Questions:
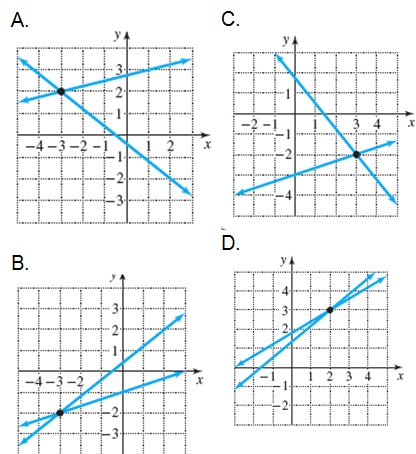
The graphs of the following systems are given in (a) through (d). Match each system with the correct graph.
1. 5x + 4y = 7
x - 3y = 9
2. 3x - 5y = -9
5x - 6y = -8
3. 4x - 5y = - 2
3y - x = - 3
4. 4x + 5y = - 2
4y - x = 11
Solve each system by substitution. Determine whether the equations are independent, dependent, or inconsistent.
5. X = 2Y - 7
3X + 2Y = - 5
6. X -Y = 5
2X = 2Y + 14
7. 3X + Y = 2
-X - 3Y = 6
Solve each system by addition.
8. X+Y = 7
X-Y = 9
9. X-Y=12
2X+Y=3
10. 2X -Y = -5
3X+ 2Y = 3
11. 2X = 3Y + 11
7X - 4Y = 6
Solve each system by the addition method. Determine whether the equations are independent, dependent, or inconsistent.
12. 3X - 4Y = 9
- 3X + 4Y = 12
Solve each system by the addition method.
13. 1/8x + 1/4y = 5
1/16x + 1/2y = 7
14. 1.5x - 2y = -0.25
3x + 1.5y = 6.375
DQ1
Prepare to discuss your findings. Discuss the necessary steps to solve for this problem.
Which of the following equations is not equivalent to: 2x - 3y = 6?
a) 3y - 2x = 6
b) Y = 2/3x - 2
c) X = 3/2y + 3
d) 2(x-5) = 3y - 4
DQ2.
Explain how you decide whether it is easier to solve a system by substitution or addition. Please provide an example to support your finding.
DQ3.
Allocating resources. A furniture maker has a shop that can employ 12 workers for 40 hours per week at its maximum capacity. The shop makes tables and chairs. It takes 16 hours of labor to make a table and 8 hours of labor to make a chair. Graph the region that shows the possibilities for the number of tables and chairs that could be made in one week. Discuss the necessary steps to solve for this problem.
More questions:
Solve the following systems
1. 3x - y = 9
2x + y = 6
2. 5x - 2y = 20
-2x + y = -10
3. X - y = 6
x + y = 7
Graph each system of inequalities.
4. y < x + 3
y < -2x + 4
5. x + y >3
x + y >1
Solve the inequality and state the solution set using interval notation
6. 3 - 2x < 9
Solve each equation or inequality
7. | 4x - 3 | = 1
Solve each equation or inequality
8. | 3(x + 2) - 4 | = 1
9. Graph the solution set of the compound inequality x < 2 or x = -2
10. Determine which of the ordered pairs (3, 2), (-3, 4), (-1, -5), and (1, -6) satisfy the compound inequality.
Y < -2x and y > x
11. Single women. The percentage of women in the 20-24 age group who have never married went from 55% in 1970 to 73% in 2000 (Census Bureau, www.census.gov). Let 1970 be year 0 and 2000 be year 30.
a) Find and interpret the slope of the line through the points (0, 55) and (30, 73).
b) Find the equation of the line in part (a).
c) What is the meaning of the y-intercept?
d) Use the equation to predict the percentage in 2010.
e) If this trend continues, then in what year will the percentage of women in the 20-24 age group who have never married reach 100%?
12. Direct deposit. The percentage of workers receiving direct deposit of their paychecks went from 32% in 1994 to 60% in 2004 (www.directdeposit.com). Let 1994 be year 0 and 2004 be year 10.
a) Write the equation of the line through (0, 32) and (10, 60) to model the growth of direct deposit.
b) Use the accompanying graph to predict the year in which 100% of all workers will receive direct deposit of their paychecks.
c) Use the equation from part (a) to predict the year in which 100% of all workers will receive direct deposit.
13. Age at first marriage. The median age at first marriage for females increased from 24.5 years in 1995 to 25.1 years in 2000 (U.S. Census Bureau, www.census.gov). Let 1995 be year 5 and 2000 be year 10.
a) Find the equation of the line through (5, 24.5) and (10, 25.1).
b) What do x and y represent in your equation?
c) Interpret the slope of this line.
d) In what year will the median age be 30?
e) Graph the equation.
14. Basal energy requirement. The basal energy requirement B is the number of calories that a person needs to maintain the life process. For a 28-year-old female with a height of 160 centimeters and a weight of 45 kilograms (kg), B is 1300 calories. If her weight increases to 50 kg, then B is 1365 calories. There is a linear equation that expresses B in terms of her weight w. Find the equation and find the basal energy requirement if her weight is 53.2 kg.