Assignment:
Problem 1 : 1D Kinematics : Relative Motion
The diagram shows a snapshot at time t=0 of the two balls (Red and Green) on a collision course. At t= 0, the balls are separated by a distance D=12m. The Green ball moves with a constant velocity VG= 6 m/s due east, while the red ball moves with constant velocity (VR) =2 m/s due west.
(a) Take the origin to be the position of red ball and positive direction to the east. Determine the equation for the displacement (x) of green ball with respect to time (t) in the reference frame of red ball?
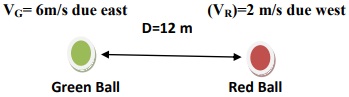
(b) Plot the equation for the displacement of the Green ball in a graph up to the scale?
Problem 2: Relative Motion: Two Swimmers
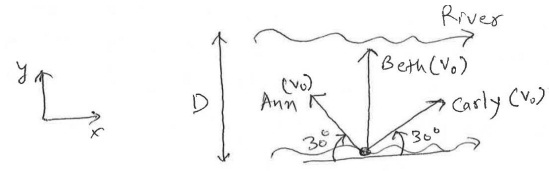
Three Swimmers can swim equally fast relative to the stream of water. They have a race to see who can swim across a river in the least amount of time.
Relative to the water,
(a) Beth swims perpendicular to the flow,
(b) Ann swims upstream at 30o, and
(c) Carley swims downstream at 30o
Calculate the velocities of all the 3 girls crossing the river assuming their initial velocity (v0) as 2 m/s? Who gets across the river first ?
Problem 3: Newton's Laws of Motion: Free body Diagram
A block of mass (m2) slides on a table pulled by a string attached to a load (m1) hanging over a frictionless pulley over the side as shown. The coefficient of kinetic friction between the sliding block and table is [µk]. Develop a suitable free body diagram (FBD) that represents the dynamics of the mass.

(i) Find the acceleration (a1) of load (m1) and acceleration (a2) of mass (m2) respectively. Show your work in detail.
(ii) Find the tension (T1) in the string pulling the load (m1), and tension (T2) in the string attached to the mass (m2) on the table.
Problem 4: Hanging Sculpture
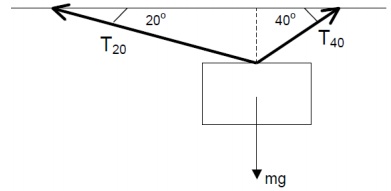
You are part of a team to help design the atrium of a new building. Your boss, the manager of the project, wants to suspend a 10-kg sculpture high over the room by hanging it from the ceiling using thin, clear fishing line (string) so that it will be difficult to see how the sculpture is held up. The only place to fasten the fishing line is to a wooden beam which runs around the edge of the room at the ceiling.
The fishing line that she wants to use is known to be able to support a vertically hanging mass of 10 kg, so she suggests attaching two lines to the sculpture to be safe. Each line would come from the opposite side of the ceiling to attach to the hanging sculpture. Her initial design has one line making an angle of 20o with the ceiling and the other line making an angle of 40o with the ceiling. She knows you are taking physics, so she asks you if her design can work.
Problem 5: Emergency Stop
Your friend has been hired to design the interior of a special executive express elevator for a new office building. This elevator has all the latest safety features and will stop with an acceleration of g/3 in the case of an emergency. The management would like a decorative lamp hanging from the unusually high ceiling of the elevator. He designs a lamp which has three sections which hang one directly below the other. Each section is attached to the previous one by a single thin wire, which also carries the electric current. The lamp is also attached to the ceiling by a single wire. Each section of the lamp weighs 7.0 N.
Because the idea is to make each section appear that it is floating on air without support, he wants to use the thinnest wire possible. Unfortunately the thinner the wire, the weaker it is. Since he knows that you have taken a course in physics, he asks you to calculate the force on each wire in case of an emergency stop.

Problem 6: Friction: Introductory and Time-dependent
1. A block of mass M = 5 kg slides on a horizontal table. The kinetic coefficient of friction between the block and the table is µk = 0.38. If the initial speed of the block is 8 m/s, how many seconds does it slide before stopping?
2. A block of mass M = 15 kg is pulled across a horizontal table by a string. The kinetic coefficient of friction between the block and the table is m = 0.69. If the speed of the block is constant at 2 m/s, what must the tension in the string be?
3. A block of mass M is released from rest on an incline of length L which makes an angle (θ) with the horizontal. The block reaches the end of the incline in time T. Show how the coefficient of kinetic friction (µk) can be determined from the measurement of time T.
4. A block of mass M rests on an incline of length L which makes an angle θ with the horizontal. The angle is slowly increased until the block starts to move. Let the angle at which the block starts to move be θs. Show that how the coefficient of static friction (µk) can be determined from the measurement of θs.
Problem 7: Exit Ramp
On a trip to the Colorado Rockies, you notice that when the freeway goes steeply down a hill, there are emergency exits every few miles. These emergency exits are straight ramps which leave the freeway and are sloped uphill. They are designed to stop runaway trucks and cars that lose their brakes on downhill stretches of the freeway even if the road is covered with ice. You are curious, so you stop at the next emergency exit to take some measurements.
You determine that the exit rises at an angle of 10o from the horizontal and is 100m long. What is the maximum speed of a truck that you are sure will be stopped by this road, even if the frictional force of the road surface is negligible?