The following 20 multiple choice questions must need to be answered. It should be accurate and correct.
Question 1:
The Australian Medical Association released a Position Statement on Men’s Health in 2005 which reported the results of a survey conducted for a program called “Bettering the Evaluation and Care of Health (BEACH)”. Based on the survey results for visits to GPs, the conclusions reached were that 76% of Australian males visited their GP during the last 12 months compared to 87% of Australian females. Which of the following choices best describes this type of statistical problem?
(a) Statistical inference
(b) Probability
(c) Descriptive statistics
(d) Parameter analysis
(e) The situation described is not a statistical problem
Question 2:
Ten runners took part in a final of the 100m sprint. While all of them were able to complete the race in less than 12 seconds, half of them completed the race in less than 11 seconds. What broad area of statistics does this problem belong to?
(a) Statistical inference
(b) Probability
(c) Descriptive statistics
(d) Parameter analysis
(e) The situation described is not a statistical problem
Question 3:
The following information was collected from students upon exiting the CQU Bookshop:
• CQU Program Enrolment code (ie. PROGRAM) eg. CQ18
• Amount of money spent on books (ie. AMOUNT) eg. $120.50
• Customer service rating (ie. RATING) where: 1 = very poor, 2 = poor, 3 = average, 4 = good and 5 = very good
The scales of measurement for PROGRAM, AMOUNT and RATING are best described as:
(a) ordinal, ratio, ordinal
(b) nominal, ordinal, ordinal
(c) nominal, interval, nominal
(d) nominal, ratio, ordinal
(e) nominal, ratio, nominal
Question 4:
In Term 1 2015 the percentage of STAT11048 students enrolled on each campus or studying by distance is given in the table below.
Campus or Distance Percentage (%)
Brisbane 12
Distance 57
Melbourne 7
Rockhampton 11
Sydney 13
Which of the following graphical procedures is best suited to the graphical presentation of this information?
(a) Bar Chart
(b) Grouped Bar Chart
(c) Line Graph
(d) Combination graph
(e) Pie Chart
Question 5:
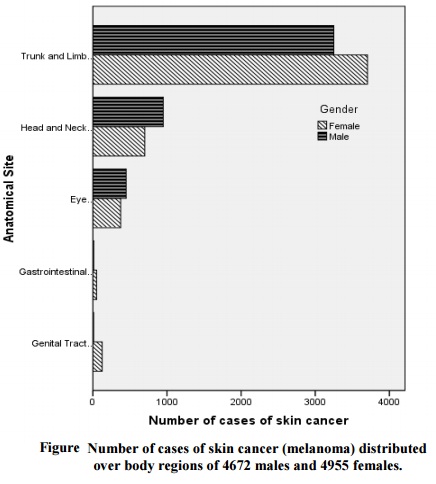
According to information obtained from the sample data shown in Figure, which of the following statements is NOT correct?
(a) Males outnumbered females only in the "head and neck" and "eye" categories.
(b) For both females and males, the most frequent site (part) of the anatomy (body) for melanomas is "trunk and limb".
(c) There were more males with trunk and limb melanomas than in all the other anatomical categories (parts of the body) combined.
(d) If 4600 males and 2000 females had been randomly sampled, the graph would have changed so much that "trunk and limb" would show more males than females.
(e) If we are interested in comparing the skin cancer incidence rates between males and females at each anatomical site, it is better to present actual frequency counts rather than relative frequency for each gender.
Questions 6 to 8 refer to the following information.
The Food & Nutrition Board of the National Academy of Sciences states that the recommended daily allowance of iron is 18 mg for adult females under the age of 51. The amount of iron intake, in milligrams, during a 24 hour period, for a sample of 45 females under the age of 51, is as follows:
15.0 18.1 14.4 14.6 10.9 18.1 18.2 18.3 15.0
16.0 12.6 16.6 20.7 19.8 11.6 12.8 15.6 11.0
15.3 9.4 19.5 18.3 14.5 16.6 11.5 16.4 12.5
14.6 11.9 12.5 18.6 13.1 12.1 10.7 17.3 12.4
17.0 6.3 16.8 12.5 16.3 14.7 12.7 16.3 11.5
Construct a frequency distribution using "6 mg to less than 8 mg" as the first class. Use equal class intervals or widths.
Question 6:
What is the relative frequency for the "14 mg to less than 16 mg" class?
(a) 0.8
(b) 9
(c) 0.2
(d) 27
(e) 0.156
Question 7:
What is the cumulative frequency for the "10 mg to less than 12 mg" class?
(a) 7
(b) 9
(c) 20
(d) 2
(a) 18
Question 8
What is the cumulative relative frequency (%) for the "16 mg to less than 18 mg" class?
(a) 20%
(b) 36%
(c) 97.78%
(d) 60%
(e) 80%
Question 9;
Construct the ogive and use it to show that the first quartile (ie. 25%) of the daily iron intake distribution is best approximated by:
(a) 11 mg
(b) 11.5 mg
(c) 12 mg
(d) 12.5 mg
(e) 13 mg
Question 10:
A biologist measured a sample of 25 maple trees on a university campus. The trunk circumferences (from 33cm to 97cm) have been arranged into the stem-and-leaf display shown below.
Stem Leaves
3 3
4 9
5 1 5 7
6 0 5 6 7 8
7 2 3 4 5 7 8 9 9
8 3
9 1 2 2 3 4 7
What proportion of the maple trees had a trunk circumference greater than 76cm?
(a) 0.40
(b) 0.60
(c) 0.44
(d) 0.52
(e) 0.56
Questions 11 and 12 use the following information:
A sample of 18 employees at a company is monitored to see how long they break for lunch on a particular day. The following data is the lunch break (in minutes) for each worker:
25 25 32 45 22 34 56 51 35
30 33 38 44 31 48 36 26 36
Question 11:
The standard deviation (in minutes) for this data is:
(a) 91.60
(b) 10.07
(c) 9.57
(d) 9.54
(e) 9.27
Question 12:
The coefficient of variation (%) of the data is:
(a) 26.54%
(b) 25.9%
(c) 35.94%
(d) 247.6%
(e) cannot be calculated for a sample
Questions 13 and 14 use the following information:
The following frequency table gives the number of road fatalities in South Australia in 2004.
Age Class No. fatalities
0 to less than 15 years old 6
15 to less than 30 years old 52
30 to less than 45 years old 34
45 to less than 60 years old 27
60 to less than 75 years old 13
75 to less than 90 years old 6
TOTAL 138
Question 13:
The median age (in years) of a road accident victim in South Australia in 2004 is approximately:
(a) 32.85
(b) 38.26
(c) 37.5
(d) 45.0
(e) 34.85
Question 14:
The standard deviation of the age (in years) of the 2004 population of road accident victims is approximately:
(a) 25.62
(b) 28.06
(c) 18.44
(d) 656.25
(e) 340.18
Question 15:
The new employees hired by a particular company over the last five years have a mean age of 35 years and a standard deviation of 5 years. If nothing is known about the shape of the new employee age distribution, which of the following statements must be true.
(a) Approximately 68% of new employees are between 30 and 35 years old.
(b) At least 75% of new employees are between 30 and 35 years old
(c) At least 75% of new employees are between 25 and 45 years old
(d) At least 89% of new employees are between 25 and 45 years old
(e) Approximately 95% of new employees are between 25 and 45 years old
Question 16:
A law firm plans to hire five (5) new lawyers for the coming financial year. After interviewing prospective employees, the firm decides that seven (7) men and nine (9) women qualify for the job. How many different groups of five (5) new lawyers can be hired if the firm decides to hire two (2) men and three (3) women?
HINT: Consider the similarity to the number plate examples in the Study Guide.
(a) 1764
(b) 21168
(c) 546
(d) 1260
(e) 105
Questions 17 to 19 use the following information:
The owner of a small travel business classifies telephone calls into those from existing clients or those from potential new clients. Eighty percent (80%) of all calls received are from potential new clients. Of the calls from existing clients, 60% result in a travel sale, while only 20% of calls from potential new clients result in a travel sale. A computer database is used to store information about each call.
(HINT: Construct a contingency table).
Question 17:
What is the probability that a randomly selected call (from the database) is “from a potential new client AND results in a travel sale”?
(a) 0.20
(b) 0.16
(c) 0.92
(d) 0.80
(e) 0.224
Question 18:
What is the probability that a randomly selected call is “from an existing client OR does not result in a travel sale”?
(a) 0.40
(b) 0.92
(c) 0.88
(d) 0.08
(e) 0.84
Question 19:
Given that a travel sale is made from the chosen call, what is the probability that it was initiated by a potential new client?
(a) 0.889
(b) 0.800
(c) 0.571
(d) 0.200
(e) 0.160
Question 20:
A string of Christmas tree lights contains 20 bulbs. The bulbs are wired in series such that if any light bulb fails then the whole string of lights will go dark. Each bulb has a probability of 0.02 of failing during the Christmas season and the bulbs fail independently of each other. What is the probability that the whole string of Christmas tree lights will remain bright during the Christmas season?
(a) 0.6676
(b) 0.98
(c) 0.3324
(d) 0.4
(e) 0.6