1) Describe the omitted variable bias problem. Suppose the true model is Y = β0 + β1X1 + β2X2 + u, but the researcher performs a regression of Y on X1 only. When will the slope coefficient of X1 be biased upward? Explain.
2) The following table of estimation results is obtained from statistical software. Suppose the significance level for decision-making purposes is 5 percent. Discuss whether the regressors provide any explanatory power on Y.
Dependent Variable Y Coefficients Standard Error t Stat P-value
Intercept 1.20 0.70 1.71 0.09
X1 Variable 0.52 0.29 1.80 0.07
X2 Variable -3.31 2.06 -1.61 0.11
F-statistic (overall significance) = 3.3; p-value = 0.04. Sample size=100.
3) Consider an OLS regression of Y on X. A scatterplot of squared residuals (û2) against X reveals the following pattern:
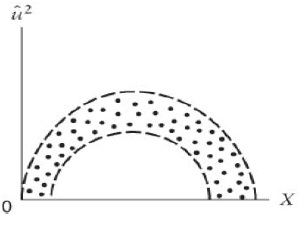
Explain how the above pattern relates to CLRM assumptions and the validity of OLS estimation results obtained from statistical software.
4) You are interested in understanding the determinants of infant’s birth weight. You have access to a data set which contains variables on infant’s birth weight in grams (bwght), infant’s gender (male=1 if male, =0 if female), infant’s race (white=1 if white, =0 otherwise), father’s years of education (fatheduc), and mother’s years of education (motheduc).
a) The following estimation result by OLS is obtained:
bwght = 3178 + 86.6*male + 180.1*white;
R2=0.0218; Adjusted R2=0.0203.
Explain the results.
b) The following estimation result by OLS is obtained:
bwght = 3170 + 101.3*male + 190.4*white – 18.6*male*white;
R2=0.0218; Adjusted R2=0.0197.
Explain the coefficient of the interaction term.
c) Why is the adjusted R2 in model b smaller than that in model a?
d) How do you test that the effect of race on birth weight is equal across gender?
e) The following estimation result by OLS is obtained:
bwght = 3038 + 108.7*male + 129.4*white + 16.9*fatheduc – 2.92*motheduc.
How do you test that parents’ education has no joint effect on birth weight at all? Describe the procedures of such a test