Assignment:
Problem 1.
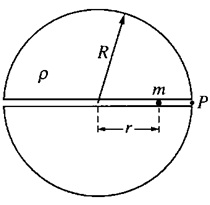
A perfectly round planet has a radius of R and a uniform density of p. Suppose a narrow tube were bored through the planet directly through it's center, as shown in the above picture, in which a small object of mass m could move freely under the influence of gravity. Let r be the distance of the mass from the center of the planet as a function of time.
F = GmMp/r2 ρ = Mp/VpVsphere = 4/3 π r3
a. Show mathematicallythat this is an example of Simple Harmonic Motion? Justify your answerusing the provided equations and demonstrating your knowledge of the conditions needed.
b. What is the angular velocity ω of the small mass at a distance r < R from the center of the planet in terms of π, G,p
The mass is dropped into the tunnel from rest at point P at the planet's surface.
c. Determine the speed of the small mass when it reaches the center of the planet in terms ofthe density of the planet ρ, the radius R and constants.
d. Fully describe the subsequent motion of the small mass from the time it reaches the center of the planet. (What happens after it reaches the center and why)
e. Write an equation that could be used to calculate the time it takes the small mass to move from point P to the center of the planet. Be specific to this problem.
f. Would your answer to part e change if you dropped the small mass from ½ the distance from point P to the center? Justify your answer.
Problem 2.
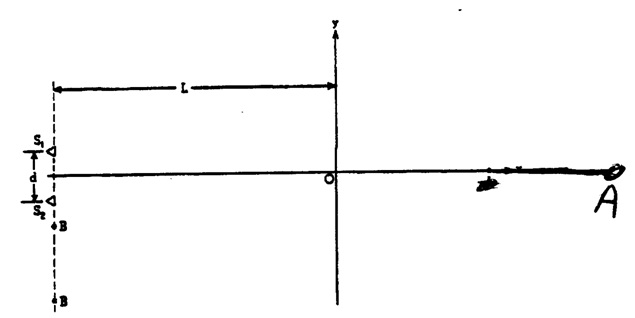
Two sound sources, S1 and S2, a distance d = 4m apart as shown in the picture above. Both sources vibrate in phase and emit auditory waves of equal amplitude and wavelength λ . L = 40 m
a. A personat point O, 40 meters away hears a sound intensity of I = 2 x 10-4 W/m2. What db level is this? (I0 = 1 x 10-12W/m2)
b. When she moves to point A, exactly 80 meters away from the speakers (twice as far away),
i. what is the Intensity at A.
ii. What decibel level is it at A?
c. Assume that d = 4 meters and that the speed of sound is 360 meters per second. Find the lowest speaker frequency which will yield the minimum sound intensity (that means quiet) along the line BB'.
d. About how far away from point O on line OY would she have to move in order to hear a maximum using the 8th harmonicof what you found in part C above?

Problem 3. This cool apparatus is set up similar to the one we used in our lab. The tuning fork has a frequency 120 Hz. Ahanging mass M is creating the tension needed to create the standing wave pattern shownin the picture above. The magnitude of M is such that the standing wave pattern has four antinodes or "loops." The string from the tuning fork to the point where the string touches the top of the pulley has a length of 1.20 m. The linear density of the string is 1.0 x 10 4 kg/m, and remains constant throughout.
a. What is the wavelength of the standing wave pattern?
b. Calculate the speed of the transverse waves along the string.
c. What is the magnitude of the mass M?
d. The speed of waves along the string increases with increasing tension in the string. Should the magnitude of M be increased or decreased in order to double the number of antinodes in the standing wave pattern. Justify your answer.
e. If a point on the string at an antinode moves a total vertical distance of 4 cm during one complete cycle, what is the amplitude of the standing wave?
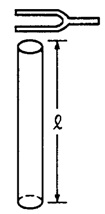
Problem 4. A plastic tube of length l open at both ends is shown in the above picture. You strike a tuning fork of frequency f o, place it near one end of the tube and it causes the air in the tube to "sing along" or resonate at its fundamental frequency like we did in our lab (except it's open at both ends for now) .
Express your answers in terms of l and/or fo
a. Find the wavelength of the standing wave in the tube in terms of l.
b. Determine the speed of sound in the air inside the tubein terms of l and/or fo
c. Determine the next higher frequency at which this air column would resonatein terms of fo.
Note: Figure not drawn to scale
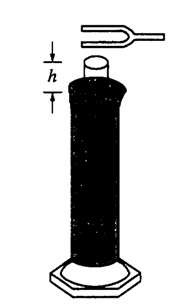
The same plastic tube is dunked in a large, graduated cylinder filled with water The tube is slowly raised out of the water and the same tuning fork, vibrating with frequency fo, is held a fixed distance from the top of the tube. (Now this is just like your lab. The water top acts like a closed end)
d. Determine the height h of the tube above the water when the air column resonates for the first time. Express your answer in terms of l
Problem 5. In MKS units, a wave on a wire is described by the formula Y(x,t) = 0.037 sin (12 x + 64 t)
a. What is the wave's amplitude?
b. What is the wave's wavelength?
c. What is the wave's period?
d. What is the wave's velocity (a vector-so state the direction)?
e. If the wave is reflected from a fixed end at x = 0, write the expression for the reflected wave. (not the standing wave).