1. Derive a demand curve.
James is at the University Book Store. He needs some ring binders and highlighter pens. The following table
shows the total utility that James gets from using ring binders.
Table 1
Number of ring binders Total Utility Marginal Utility
0 0 ---
1 32
2 60
3 80
4 90
a. Calculate the marginal utility of using each unit of ring binders.
The following table shows the marginal utility that James gets from using each unit of highlighter pens.
Table 2
Number of highlighter pens Total Utility Marginal Utility
0 0 ---
1 15
2 14
3 13
4 12
5 11
6 10
7 9
8 8
b. Complete by calculating his total utility.
c. Suppose that James has an income of $24 that he can spend on buying ring binders and highlighter pens. The price of one ring binder is $8 and the price of one highlighter pen is $4. If he spends all of his income buying ring binders and highlighter pens, which consumption bundles are affordable to him? Answer this question by completing
below.
Table 3
Price of a Price of a Number of Number of Income
ring binder highlighter pen ring binders highlighter pens
$8 $4 0 $24
$8 $4 1 $24
$8 $4 2 $24
$8 $4 3 $24
d. From
to , find the number of ring binders and highlighter pens that James consumes to maximize his utility under the budget constraint described in (c). (Hint: Diamond-Water paradox)
e. Suppose that the price of a highlighter pen decreases to $2. Holding everything else constant, how many ring binders and highlighter pens will James choose to consume? (Hint: It will be convenient to complete following table.)
Price of a Price of a Number of Number of Income
ring binder highlighter pen ring binders highlighter pens
$8 $2 0 $24
$8 $2 1 $24
$8 $2 2 $24
$8 $2 3 $24
f. Assume that James’ demand function for highlighter pens is linear. Then from the results in (d) and (e), derive his demand function for highlighter pens.
2. Budget Lines and Indifference Curves.
A. Suppose Paul has an income of $12 to buy sandwiches and coffee. The price of a sandwich is $2 and the price of a cup of coffee is $3.
a. Draw Paul’s budget line (BL1) using the information above. Put sandwiches on the x-axis and coffee on the y-axis. Derive the equation for his budget line. It is known that Paul consumes 3 sandwiches and 2 cups of coffee under this budget constraint. Label this consumption bundle as point A and draw the bowed-inward indifference curve, IC1, passing through the point A.
b. Suppose that the price of a sandwich increases to $3. Holding everything else constant, draw the new budget line (BL2) on the same graph you used in (a). Derive the equation for the new budget line. It is known that Paul will choose 2 sandwiches and 2 cups of coffee under the new budget constraint. Label this point as point B and draw the bowed-inward indifference curve, IC2, passing through the point B. (Caution: Indifference curves should not cross each other!)
c. There are two reasons why Paul decreases his demand for sandwiches when the price of sandwiches increases. (1)The first reason is because sandwiches become relatively expensive compared to coffee; he will substitute his demand for sandwiches for demand for coffee which is relatively cheaper. (2)The second reason is because his real income decreases due to the increase in the price of a sandwich; he can’t afford the initial consumption bundle after the increase in the price of a sandwich. Which one is the explanation for income effects and which one is the explanation for substitution effects?
d. Now we want to separate these two effects and see how demand for sandwiches changes due to each effect. Draw an imaginary budget line, say BL3, which is parallel to BL2 and tangent to IC1. And illustrate the substitution and income effects in sandwich consumption due to the change in the price of sandwiches on your graph.
B. Michael likes to eat pizza. He receives 2 units of utility for each slice of pepperoni pizza and 3 units of utility for each slice of sausage pizza.
a. Draw the indifference curve corresponding 18 units of utility for Michael. Put sausage pizza on the x-axis and the pepperoni pizza on the y-axis.
b. Suppose that each slice of both pepperoni and sausage pizza costs $3. Furthermore, assume Michael has an income of $18 to spend on pizza. Draw the budget line (BL1) using this information. Derive the equation for the budget line. Under this budget constraint, how many slices of sausage and pepperoni pizza does he consume to maximize his utility? Label this consumption bundle as point A and draw the indifference curve (IC1) passing through the point A.
c. Suppose that the price of a slice of sausage pizza increases to $6. Holding everything else constant, draw the new budget line (BL2) on the same graph you used in (b). Derive the equation for the new budget line. Under the new budget constraint, how many slices of sausage and pepperoni pizza does he consume to maximize his utility? Label this consumption bundle under the new budget constraint as point B and draw the indifference curve (IC2) passing through the point B.
d. Draw an imaginary budget line (BL3) parallel to the new budget line (BL2) and make it touch the initial indifference curve (IC1) at the lowest possible income level. Calculate and show the income and substitution effects in the consumption of sausage pizza due to the change in the price of sausage pizza. (Caution: There will be lots of lines. Don’t be confused! And it is very important to verify the slope of each line and draw it appropriately.)
C. Nathan likes to eat hamburgers he makes. He always uses two patties and one tomato to make one hamburger. However, he doesn’t like patties or tomatoes by itself. That is, 2 patties and 2 tomatoes are as good as 2 patties and 1 tomato to Nathan.
a. Draw the indifference curve corresponding 1 hamburger to Nathan. Put tomatoes on the x-axis and patties on the y-axis.
b. Suppose that each patty costs $1 and each tomato also costs $1. Furthermore, assume Nathan has an income $15 to spend in making hamburgers every week. Draw the budget line (BL1) using this information. Derive the equation for the budget line. How many patties and tomatoes does he consume to maximize his utility? Label this consumption bundle as point A and draw the indifference curve (IC1) passing through the point A. (Hint: At the optimal consumption bundle, he always chooses 1 tomato and 2 patties rather than 2 tomatoes and 2 patties even though these two consumption bundles give him one hamburger. Why?)
c. Suppose that the price of tomatoes decrease to $.5. Holding everything else constant, draw the new budget line (BL2). Derive the equation for the new budget line. How many patties and tomatoes does he consume to maximize his utility under the new budget constraint? Label this new consumption bundle as point B and draw the indifference curve (IC2) passing through the point B.
d. Draw an imaginary budget line (BL3) parallel to the new budget line (BL2) and make it touch the initial indifference curve (IC1) at the lowest possible income level. Calculate and show the income and substitution effects in the consumption of tomatoes due to the price change in tomatoes.
3. Production Cost:
The following table gives cost information for a firm. Assume that labor is paid a constant wage and capital is paid a constant price, i.e., our firm is a price-taker both in the labor and capital markets.
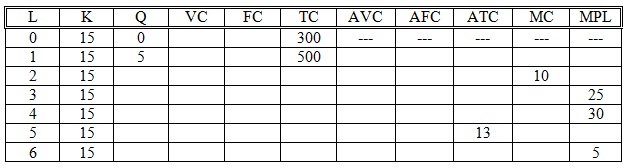
a. Complete the above table with specific numbers.
b. At what level of output is ATC at its minimum?
c. At what level of labor usage does the law of diminishing returns first occur?
d. At what level of output does marginal cost attain its minimum?
e. When output is equal to zero units, why does the firm still incur costs in the short run? Explain your answer.
4. Perfect Competition:
The figure below shows three curves, MC, ATC and AVC, for the firm in a perfectly competitive market. In this market, the price of the good is $4. Use the information given in the figure to answer the following questions.
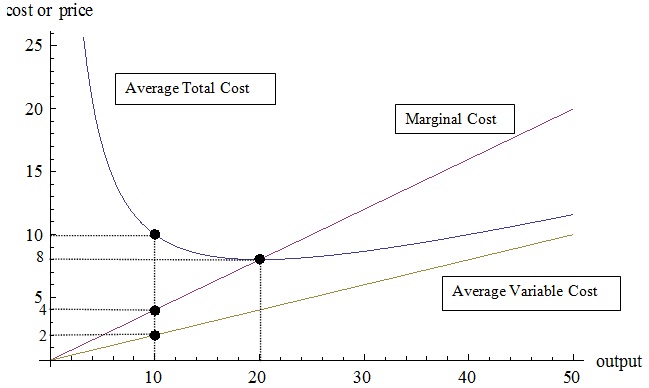
a. In the short run, will the firm shut down the production when price equals $4? Explain your answer.
b. If the firm does not shut down in the short run when price equals $4, will the firm make a positive economic profit or a negative economic profit? What is the value of the firm's economic profit when price equals $4?
c. If the firm does not shut down in the short run when price equals $4, what will be the firm's production level? Calculate the firm's fixed cost and variable cost at this level of production.
d. What is the break-even price for the firm? What is the shut-down price for the firm?
1414317
Questions
Answered
Start Excelling in your courses, Ask a tutor for help and get answers for your problems !!
ask Question