Discuss the below:
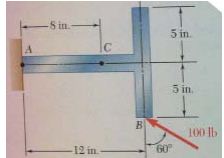
Q1) Compute the moment of the 100-lb force about A, (a) by using the definition of the moment of a force, (b) by resolving the force into horizontal and vertical components, (c) by resolving the force into components along AB and in the direction perpendicular to AB.
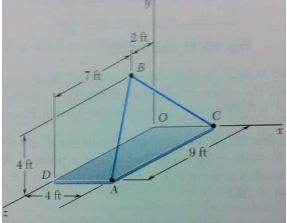
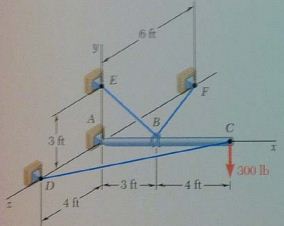
Q2) Knowing that the tension in cable BC is 900 lb, determine the moment of the force exerted on the plate at C about (a) the origin of coordinates O, (b) corner D.
Q3) A force P of magnitude 25 lb acts on a bent rod as shown. Determine the moment of P about (a) a line joining points C and F, (b) a line joining points O and C.

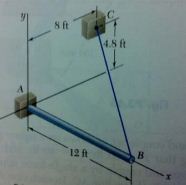
Q4) The 12-ft boom AB has a fixed end A, and the tension in the cable BC is 570 lb. Replace the force that the cable exerts at B by an equivalent force-couple system at A.
Q5) A 7-ft boom is held by a ball and socket at A and by two cables EBF and DC; cable EBF passes around a frictionless pulley at B. Determine the tension in each cable.
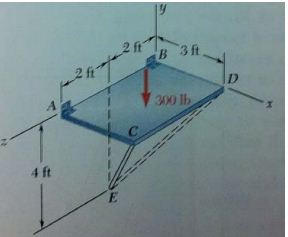
Q6) The horizontal platform ABCD weighs 60 lb and supports a 240-lb load at its center. The platform is normally held in position by hinges at A and B and by braces CE and DE. If brace DE is removed, determine the reactions at the hinges and the force exerted by the remaining brace CE. The hinge at A does not exert any axial thrust.
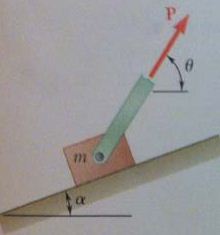
Q7) A block of mass m = 20 kg rests on a rough plane as shown. Knowing that alpha = 25 degree and mu_s = 0.20, determine the magnitude and direction of the smallest force P required (a) to start the block up the plane, (b) to prevent the block from moving down the plane.