There are two questions in the problem set
Question 1:
An economy’s technology is described by the following Cobb-Douglas production function.
Y = K0.50N0.50
Where, Y is the real GDP and K and N are the amounts of capital and labor. We have the following information about this economy:
Labor grows at an annual rate of 2% per year → n = 0.02
Annual depreciation rate of capital is 3% → d = 0.03
Saving rate is 25% → s = 0.25
a. Find the steady state levels of k and y. Show this case in a nice and readable Solow diagram (the graph must be fully labeled with the values of k*, y*, (d + n)×k* and s×y*.
b. If “this year” capital per worker was k = 9, at what rate would it change from “this year” to “the next year” (in other words, calculate Δk/k)? What if k was equal to 16? Show these cases in a Solow diagram.
From this exercise, what do you conclude about the relationship between k and Δk/k? What is the economics behind this relationship?
Hint: Even though we did not prove it in the class, the difference between the actual investment per worker (s×y) and the one required to keep k constant ((d + n)×k)) equals the change in k. Specifically, ?k=sy-(d+n)k
c. Suppose that “this year” we are in steady state with K = 2,500 and Y = 500. Assuming steady state, if you look at this economy “next year”, what will you find for the values of K, N, Y, k, and y?
Question 2:
An economy’s technology is described by the following Cobb-Douglas production function.
Y = AK0.25N0.75
Where, Y is the real GDP and K and N are the amounts of capital and labor. Currently the technology parameter equals A = 16. We have the following additional information about this economy:
Labor grows at an annual rate of 1% per year → n = 0.01
Annual depreciation rate of capital is 4% → d = 0.04
Saving rate is 20% → s = 0.20
a. Find the steady state levels of k and y. For the sake of argument let’s suppose that this solution pertains to the year 2016. Show this case in a nice and readable Solow diagram (the graph must be fully labeled with the values of k*2016,y*2016,(d+n)k*,and s×y*2016
b. Technology improves by 5% from 2016 to 2017. Find the values of k*2017,y*2017.Show all the shifts with the 2016 and 2017 equilibrium values in a Solow diagram.
c. Find the rates of growth of the steady-state capital per worker and GDP per worker. Show your work.
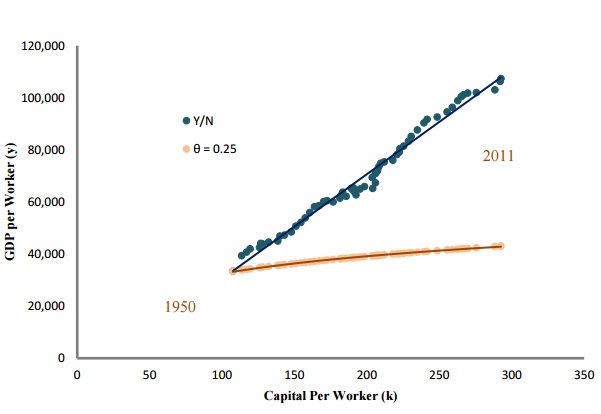
d. Now here is the cool part. Consider the graph below that you have seen several times before.It shows that, unlike what Cobb-Douglas predicts, the relationship between k and y for the U.S. was more or less linear (no diminishing returns to capital).
One way to resolve this conflict was to argue that improvements in technology (know-how) had caused the function to shift up over years so that the actual (blue) points observed in the graph are the steady-state k-y pairs lying on different production functions. Assume that technology improves one more time in 2018 by the same 5%.
Find the values of k*2018 and y*2018. Do the three k*-y* pairs for 2016, 2017, and 2018 lie on a straight line? If yes, what is the equation of this line?