Question 1: This question is focused on a very general math review. Please make sure to show all your work and not just a final answer for each question.
a. Suppose you are told that the points (x, y) = (10, 20) and (x, y) = (20, 10) sit on a straight line. What is the equation for this line in slope-intercept form?
b. Suppose you are told that the points (x, y) = (10, 5) and (x, y) = (20, 20) sit on a straight line. What is the equation for this line in slope-intercept form?
c. You are told that a straight line has slope of -5 and that the point (x, y) = (10, 10) sits on this line. What is the y-intercept for this line?
d. You are told that a straight line has slope of -5 and that the point (x, y) = (10, 10) sits on this line. What is the x-intercept for this line?
e. You are given the equation y = 10 + 2x and told that at every y value the x value is now 20 units larger. What is the equation for this new line?
f. You are given the equation y = 10 + 2x and told that at every x value the y value is now 10 units smaller. What is the equation for this new line?
Question 2: This question is meant to review some more math concepts. Please show all your work and not just your final answer.
a. Suppose that Jack has 150 books and he goes out this weekend and purchases an additional 30 books. What is the percentage change in the number of books that Jack owns?
b. Suppose that Chem 103 has two midterms and a final. Each midterm is worth 25% of the final grade and the final is worth 50% of the final grade. Josie currently has a 80 on the first midterm and a 72 on the second midterm. To earn a B in the class Josie needs to have a weighted average of 88 in the class. What is the minimum final exam score Josie can earn and still get a B? Assume that all three exams are 100 point exams.
c. Let’s return to Jack. Jack now owns 180 books but unfortunately a fire at Jack’s apartment destroys 30 books. What is the percentage change in the number of books Jack owns given this information?
d. Ellen’s teacher in Physics is giving three exams over the course of the semester. The first two exams are each worth 30% of the final grade while the third exam is worth 40% of the final grade. Ellen’s teacher has confused Ellen a bit by giving exams with different amounts of points even though each exam will have the given weights based on the exams being 100 point exams. Ellen made a 20 out of a possible 40 points on the first exam; a 40 out of a possible 50 points on the second exam; and a 45 out of 50 points on the final exam. On a 100 point scale what is Ellen’s weighted average in this class?
Question 3: Use the following diagram of a production possibilities frontier for Macroland to answer the following set of questions. Assume that Macroland produces only two types of goods, capital goods (K) and consumer goods (C).
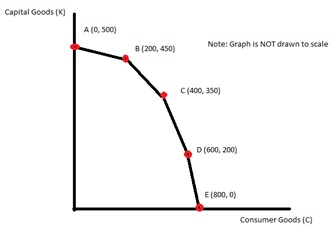
a) What is the opportunity cost of producing 50 more units of capital goods if the economy of Macroland is currently producing at point B?
b) What is the opportunity cost of producing 50 more units of consumer goods if the economy of Macroland is currently producing at point B?
c) What is the opportunity cost of producing 1 more unit of capital goods if the economy of Macroland is currently producing at point C?
d) What is the opportunity cost of producing 1 more unit of consumer goods if the economy is currently producing at point D?
e) Suppose that there is a technological improvement in producing consumer goods that results in twice as many units of consumer goods being produced from the available resources in Macroland. Draw the new production possibility frontier for Macroland given this change. Assume that there are no changes in technology with regard to capital good production.
Question 4. Amazonia and Noblia are two countries that each produce zippers (Z) and hats (H). From its available resources and technology Amazonia can produce 100 zippers and 0 hats or 0 zippers and 200 hats or any combination of zippers and hats that sits on Amazonia’s linear PPF. From its available resources and technology Noblia can produce 50 zippers and 0 hats or 0 zippers and 50 hats or any combination of zippers and hats that sits on Noblia’s linear PPF.
a. On two separate graphs draw the PPF for Amazonia and Noblia. Measure zippers on the vertical axis and hats on the horizontal axis.
b. Write an equation in slope-intercept form for the PPF for Amazonia.
c. Write an equation in slope-intercept form for the PPF for Noblia.
d. What is Amazonia’s opportunity cost of producing 1 hat? What is Noblia’s opportunity cost of producing 1 hat? Which country has the comparative advantage in producing hats?
e. What is Amazonia’s opportunity cost of producing 1 zipper? What is Noblia’s opportunity cost of producing 1 zipper? Which country has the comparative advantage in producing zippers?
f. Draw the joint PPF for Amazonia and Noblia. On this graph make sure you identify the coordinates of any endpoint as well as the coordinates for any “kink” point.
g. What is the range of trading prices that are acceptable to both countries for 10 zippers?
h. What is the range of trading prices that are acceptable to both countries for 5 hats?
Question 5. Joe and Sue are the only labor in the community of Dover. They both use their labor to rake leaves in yards or mow grass in yards. The yards in Dover need both raking and mowing on a weekly basis, so there is never a shortage of yards to be raked or mowed. Furthermore, all the yards in Dover are identical. It takes Joe 2 hours of labor to rake one yard and 1 hour of labor to mow one yard; it takes Sue 3 hours of labor to rake one yard and 4 hours of labor to mow one yard.
a. Take the provided information and arrange it in the following table:
Hours of Labor to Rake One Yard Hours of Labor to Mow One Yard
Joe
Sue
b. In order to construct the PPF for Joe or for Sue you need to know the hours of labor that they have available for these activities. Suppose our main goal on this problem is to identify who has the comparative advantage in the production of each good and what the acceptable range of trading prices is. Given this goal how would you determine an amount of labor hours available to Joe and Sue for this analysis?
c. Suppose Joe and Sue each has 120 hours of labor available to devote to raking and mowing. Draw two graphs: in the first graph depict Joe’s PPF for raking and mowing and in the second graph depict Sue’s PPF for raking and mowing. Measure yards raked (R) on the vertical axis and yards mowed on the horizontal axis (M).
d. Who has the comparative advantage in raking yards? Explain your answer making reference to the opportunity cost of production.
e. Who has the comparative advantage in mowing yards? Explain your answer making reference to the opportunity cost of production.
f. Draw the joint PPF for Joe and Sue based on their each having 120 hours of labor to devote to raking and mowing. Identify the coordinates of the intercepts as well as any kink in the joint PPF.
g. What is the acceptable range of trading prices for 10 raked yards?
h. What is the acceptable range of trading prices for 20 mowed yards?