Problem 1: Small Open Economy Coconut Island, a small economy, is closed to capital �flows and international trade. The nation is holding a democratic election. There are two candidates for President, and each has proposed di�erent domestic and foreign economic policies. Assume that this small op en economy can be described by the set of equations provided b elow. Recall that r � denotes the world equilibrium real interest rate
r* = 0.08
Y = 6000
C = C(r, Y-T) = 0.8(Y-T) - 1000r + 500
I = I(r) = 3790 - 25000r
Y = C + I + G + NX
Candidate 1 b elieves that the economy should remain closed, the government should collect 300 in taxes and run a balanced budget.
Candidate 2 b elieves that the country should allow international trade and the government should collect 300 in taxes and spend 500.
1.1 For each Presidential candidate, what would be the level of public savings if their proposed policies were enacted?
1.2 For each Presidential candidate, what would be the level of private savings if their prop osed policies were enacted? Note: for the closed economy, leave r as a variable for now.
1.3 For each Presidential candidate, what would be the level of national savings if their proposed policies were enacted?
1.4 For each Presidential candidate, what would be the domestic real interest rate if their proposed policies were enacted?
1.5 For each Presidential candidate, what would be the level of investment spending if their proposed p olicies were enacted?
1.6 For each Presidential candidate, what would be the level of net capital out�ows (NCO) if their proposed policies were enacted (recall that NCO = S - I)? Would this country be a net borrower or net lender on international fi�nancial markets?
1.7 You are an entrepreneur living in Coconut Island. Supp ose you hold the patent to a unique technology which can pro duce a unique product, and you want to borrow money to �nance the pro duction of this good. Ceteris paribus (all else equal), which presidential candidate would you support? Why?
Problem 2: Foreign Exchange Market
Let's say that there are only two countries in the world, the United States and Japan. Their currencies are traded in an op en (i.e. competitive) market without regulation or price controls. The nominal exchange rate (e) of Yen for US Dollars (¥/$US) is the equilibrium price determined in this foreign exchange (currency) market. You are given the following equations for the supply/demand of US Dollar.
Qs = - 3750 + 125e
Qd = 30000 - 100e
2.1 What is the nominal exchange rate in equilibrium (e *)?
2.2 The ratio of the price levels in the two economies is PUS/PJPN = 990/1500) ($US/¥). What is the real exchange rate of US Dollars for Japanese Yen?
2.3 Using your result from the previous part, compute the level of government sp ending in the US. You may assume the following macro economic model for the (open) US economy. Equilibrium in the world loanable funds market has been determined already, giving you r* exogenously.
r* = 0.05
Y = 2000
T = 50
I(r) = 325 - 2000r
NX(ε) = 200 - 2ε
C = 25 + 0.6(Y-T)
Y = C + I + G + NX
2.4 Draw a graph of the real foreign exchange market, plotting the real ex- change rate � versus net exports NX(ε) and net capital out�ows NCO , which should not depend on. Label you axes. Identify the equilibrium levels ε*� and NX �= NX (ε*) clearly on the graph.
Problem 3: Steady-State in the Lab or Market Assume the usual lab or market identity, which says that individuals in the labor force are either employed or unemployed.
LF = U + E
Let �Φ (Greek letter phi, for ��finding work�) denote the job-�finding rate, the proportion of unemployed individuals who �find work every month. In other words, ΦU individuals move from U to E on a monthly basis. Let �λ (Greek letter lambda, for �leaving work�) denote the separation rate, the prop ortion of employed individuals who lose their jobs every month. Similarly, �λE individuals move from E to U every month. De�ne steady-state in the labor market as a situation where the levels of unemployment and employment are constant over time. We can write the steady-state condition as follows
ΦU = λE
In words, this equation says that the �ows in and out of the groups ΦU and λE are equal; the number of individuals entering U equals the number of individuals leaving U, for example. �ΦU jobs are created and �E jobs are destroyed each month. Steady-state implies that job creation equals job destruction.
3.1 Assume that the steady-state condition �U = �E holds. Solve for the steady- state unemployment rate UR = U/LF in terms of parameters �Φ and �λ. This is also called the natural rate of unemployment.
3.2 Compute partial derivatives ∂UR/∂Φ� and ∂UR/∂λ�. These will be used in the next part for policy evaluation.
3.3 You have been hired as a lab or economist by the US Bureau of Lab or Statistics (BLS). The organization needs your help to reduce the US unemployment rate, which is currently at 9.8%. The BLS has estimated �Φ = 0.6 and �λ = 0.05 from historical data. Using your results from the previous part, which policy would be most bene�cial in terms of lowering the steady-state unemployment rate: a very small increase in Φ �or a very small decrease in �λ?
3.4 Provided that �Φ = 0.6 and ��λ = 0.05, calculate UR in steady-state.
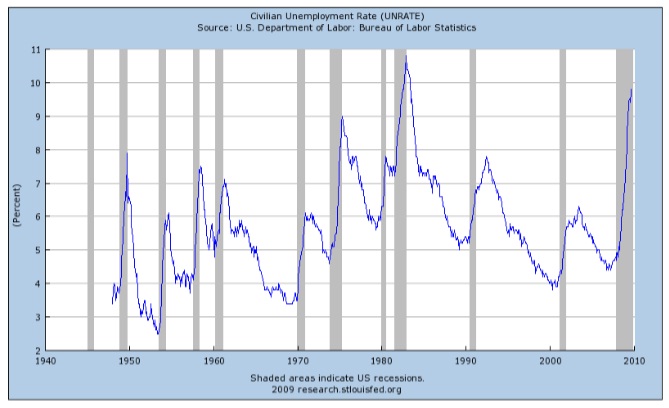
3.5 Given what you know ab out historical US unemployment rates, are the BLS estimates of �Φ and λ � reasonable? You can use the graph below to inform your response.
Computational Lab or Market Mo del (do Q3 �rst) The BLS has sent you o� to work with their latest mo del of the lab or market (see Excel �le, Q4a tab). The model can track the movements of U and E over time, if you know �Φ and λ�. Let the subscript t stand for time, which is indexed in months. Here are the initial conditions (at time t = 0 )
Φo = 0.6
λo = 0.05
Uo = 100
Eo = 920
You don't have to worry ab out this, but here is how Ut and Et change over time in the model. These equations might give you some intuition about what is going on.
Ut = (1 - Φ)Ut-1 + λEt-1
Et = (1 - λ)Et-1 + ΦUt-1
The columns have lab els U (unemployed), E (employed), LF (lab or force), UR (unemployment rate), and ER (employment rate). You can change parameters Φ� (rate of job �finding) and � λ (rate of job loss) and the model will adjust accordingly. For this problem, 0 <� t �< 30
4.1 Before you do anything in Excel, how long do you think it will take (t) for the model to reach steady-state in terms of the unemployment rate UR ? t = 2? t = 15? t = 1? Use your intuition (no wrong answer here). Explain.
4.2 Run the model by copying the formula in the t = 1 row all the way down to the t = 30 row. Complete the table. How long did the mo del actually take to converge to the steady-state unemployment rate?
4.3 Graph UR versus time. On your graph, include a horizontal line for the steady-state unemployment rate that you calculated in Q3. Is the outcome of the mo del consistent with your previous calculation of the steady-state unemployment rate? Given the initial conditions, are you converging to the steady-state value from ab ove or from b elow?
4.4 Make columns for �ΔU and �ΔE in the Excel �file. Graph ΔU and �ΔE versus time (try to do this on one graph). Given this graph, make an argument ab out how the mo del reaches the steady-state unemployment rate.
4.5 Now look at the Q4b tab. You'll see that the values of �Φ and λ �are varying over time; this is more realistic. Run the model by copying the formula in the t = 1 row all the way down to the t = 30 row. Complete the table. Graph UR versus time. Interpret your graph. Do you observe convergence to a steady-state? Why or why not?