Question 1. For each of the following pairs of coordinates (X, Y) write the equation for the straight line that contains both points. Show your work. Show how you calculated the slope of the line.
a. (X, Y) = (10, 2) and (X, Y) = (8, 4)
b. (X, Y) = (8, 5) and (X, Y) = (5, 5)
c. (X, Y) = (8, 5) and (X, Y) = (10, 10)
d. (X, Y) = (1/2, 1/4) and (X, Y) = (1/5, 1/10)
[Note: keep this as fractions and do not use a calculator: this problem is designed to help you review some basic “rules of fractions” that you will need over the course of this semester.] Show all your work on this one: no calculator shortcuts-your TA wants to see how you did the work!
e. (X, Y) = (0.2, 3.5) and (X, Y) = (2.7, 4.2) [Note: keep this in decimals and do not use a calculator: this problem is designed to help you review some basic “rules of decimals” that you will need over the course of this semester.] Show all your work on this one: no calculator shortcuts-your TA wants to see how you did the work! You will need to carry your work to at least three places past the decimal.
f. (X, Y) = (1/4, ¾) and (X, Y) = (0.5, 1.5) [Note: you will need to either convert all numbers to fractions or all numbers to decimals to answer this problem.] Show all your work on this one: no calculator shortcuts-your TA wants to see how you did the work!
Question 2. Let’s do a bit more math practice:
a. Suppose you are told that there is a straight line with slope of -4. This line includes the point (.05, 10.2). Write the equation for this line in x-intercept form. Note: do not use a calculator on this problem-we want you to practice with some decimals for a bit!
b. Suppose you are given an equation for a line: Y = 15 - 0.3X and then are told that at every Y value the X value has increased by 3 units. First draw a graph of the original equation (showing just the first quadrant values) and then sketch the new line in this same graph. Measure the Y value on the vertical axis and the X value on the horizontal axis. (Here the intent is to get you to “visualize” the two lines and their relationship to one another. You might find it fun to do this sketch using a computer drawing program like “Paint” or “Paintbrush” particularly if you have never used this kind of program.) What is the equation for this new line given this information?
c. Suppose you are given an equation for a line: X = 50 – (10/3)Y and then you are told that at every Y value the X value has increased by 3 units. What is the equation for this new line written in X-intercept form? Again, you might find it helpful to draw a sketch of the situation before you work on writing the new equation.
d. Suppose you are given an equation for a line: Y = 100 – 0.5X and then you are told that at every X value the Y value has decreased by 10 units. What is the equation for the new line written in Y-intercept form? What is the equation for the new line written in X-intercept form?
3. In this set of exercises we will combine working with equations and graphs.
a. Suppose you are given the following two equations:
Y = 10 – (1/4)X
Y = 1 + (1/8)X
Draw a graph (first quadrant only) illustrating these two lines. In your graph identify the values of any Y or X intercepts. Then, solve for the coordinate values (X, Y) where these two lines intersect. Show your work and keep all fractions as fractions as you work through the problem. Do not use a calculator or a graphing calculator: we are working on some very important skills that you will need as the course progresses!
b. Suppose you are given the following two equations:
Line 1: Y = 10 – (1/4)X
Line 2: Y = 1 + (1/8)X
But, you are also told that Line 2 has shifted to the left so that at every Y value the X value is now 16 units smaller. Write the equation for this new Line 3 and then solve for the coordinate values (X, Y) where Line 1 intersects Line 3. Show your work! Keep your answer as an improper fraction.
Question 4. Suppose you are given the following two equations:
Y = 10 – X for all values of X that are greater than or equal to 0 and all values of Y that are greater than or equal to 0 (hence, just the first quadrant values)
Y = 8 – (2/5)X for all values of X that are greater than or equal to 0 and all values of Y that are greater than or equal to 0 (hence, just the first quadrant values)
a. Draw three graphs of the first quadrant so that all three graphs are lined up in a row: here’s the image we are looking for:

In this problem it will be important that you draw “to scale”! In the first graph (the one to the far left) draw in the first line. In the middle graph draw the second line.
b. Now, we want to draw the image for the third graph. To do this, we are going to add the two lines together horizontally. To add lines together horizontally we will hold the Y value constant and then sum the X values together. So, for instance if the Y value is 2, then you would want to calculate the X value for the first equation (X1) when the Y value is set at 2 and the X value for the second equation (X2) when the Y value is set at 2. Then add these two X values together to get the new coordinate point in the third graph where (X, Y) will be (X1 + X2, 2). Be careful here: you should get two linear segments in the third graph and if you don’t get this-then you are not getting the right answer!
c. Write the two equations for the lines drawn in the third graph. For each equation provide the range of Y values for that equation and the domain of X values for that equation. Show your work.
Question 5. For each of the following statements determine whether the statement is a positive or a normative statement. Explain your answer.
a. Joe is working hard and by the end of the year his savings from his work should be sufficient to pay for a year of college tuition.
b. Susie is working hard and she ought to get more sleep than she is getting in order to function better in her day-to-day activities.
c. Mark is working hard and he ought to get straight A’s given his work effort.
d. Paul is working hard and this work effort seems to be paying off: he has been on the Dean’s List the last four semesters.
Question 6. Suppose you are given the following production possibility frontier (PPF) for the economy of Grantsville. Grantsville produces consumer goods (C) and capital goods (K) from its available resources.
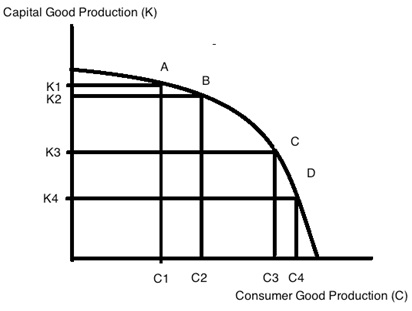
a. Given the above graph, what is the opportunity cost of moving from Point A to Point B? Make sure you identify the type of units that opportunity cost is measured in when you provide your answer.
b. Given the above graph, what is the opportunity cost of moving from Point C to Point A? Make sure you identify the type of units that opportunity cost is measured in when you provide your answer.
c. Given the above graph, does the Law of Increasing Opportunity Cost hold for capital goods? The Law of Increasing Opportunity Cost states that the opportunity cost of getting each additional unit of the good increases as you get more and more of that good. Explain your answer and provide a graph to illustrate this idea.
Question 7. Janine is taking Econ 101 and Psych 202 this semester and has figured out that she has ten hours available each week to devote to the study of economics or psychology. The table below provides information about the amount of study time she allots to each subject and the anticipated final grade she will get in each subject given this allocation of study time.
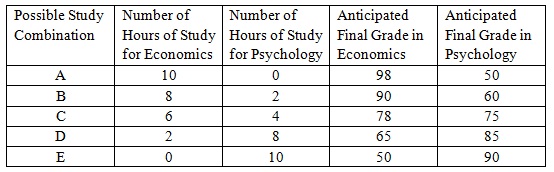
a. In a graph plot out Janine’s production possibility frontier given the above information. Measure her Economics grade on the vertical axis and her Psychology grade on the horizontal axis. Instead of using (0, 0) as the origin, use (50, 50) as the point of intersection for the two axes: do this since even if she studies zero hours for Economics or Psychology she will get a grade of 50 in the classes: not a bad assumption for many classes where the professor expects a student to be able to get approximately 50% of the material by just coming to class and hearing the lectures. Mark combinations A, B, C, D, and E on your graph and then connect these five combinations to make a PPF for Janine’s production of these two goods (the grades in Economics and the Psychology). To make this a more interesting assignment: figure out how to draw the graph using some kind of computer program: for example, Excel (here you will need to enter the data and then convert to a graph), Paint, or Paintbrush (I tend to use Paint or Paintbrush if I am making “sketches” and Excel if I want a “too-scale” drawing). (By doing this with a computer program you will build your skill sets and can add “comfortable with graphic design programs to your resume.)
b. What is Janine’s opportunity cost of changing her study habits from 8 hours of Economics study a week to 2 hours of Economics study a week? Be sure to include some kind of unit of measurement for the answer you provide.
c. Given the above information, is there an optimal allocation of study time to these two subject areas? Explain your answer.
Question 8. Consider two countries: Country X and Country Y. Both countries produce autos and shoes and the following table provides you with information about the maximum amount of autos and shoes that each country can produce if they only produce one of these goods. Both countries have linear production possibility frontiers in these two goods.

a. Given the above table, which country has the absolute advantage in the production of autos?
b. Given the above table, which country has the absolute advantage in the production of shoes?
c. Draw two graphs: in the first graph represent Country X’s PPF measuring autos on the vertical axis and pairs of shoes on the horizontal axis; in the second graph represent Country Y’s PPF measuring autos on the vertical axis and pairs of shoes on the horizontal axis. Again, you might do this on a computer graphic program (see the last question for names of some of the readily available programs).
d. What is the opportunity cost of the following (in your answer provide units of measurement):
i. One pair of shoes for Country X?
ii. One pair of shoes for Country Y?
iii. One auto for Country X?
iv. One auto for Country Y?
e. Which country has the comparative advantage in the production of autos? Explain your answer. Which country has the comparative advantage in the production of shoes? Explain your answer.
f. Draw the joint PPF for these two countries in a single graph, measuring autos on the vertical axis and pairs of shoes on the horizontal axis. Label clearly the coordinates for any “kink points”.
g. What is the acceptable range of trading prices for 10,000 pairs of shoes? Provide a number line like the one presented in class to illustrate your answer. Include the directional arrows and the Country Perspective in your number line illustration.
h. What is the acceptable range of trading prices for 5,000 autos? Provide a number line like the one presented in class to illustrate your answer. Include the directional arrows and the Country Perspective in your number line illustration.