Assignment:
A new road is to be cut through a hill, and the contractor responsible for building the road wants to know how much soil will need to be removed. A diagram of the hill and the proposed cutting is given below.
A surveyor measures the profile of the hill along the 300m length of the proposed cutting and finds that it can be described by the curve
y = -8x^4 + 56x^3 - 136x^2 + 120x
where y is the height of the hill in metres a distance x hundred metres along the path of th cutting.
(a) Copy and complete the following table, then sketch the profile of the hill for 0 <= x <= 3.
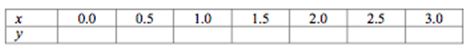
(b) To calculate the volume of soil to be removed the surveyor needs to calculate the area under the curve y along the 300m path of the cutting. Write an expression for the area under the curve as an integral.
(c) Using the expression you have given for part (b) calculate the area of the cross section of the cutting, giving your answer in square metres.
(d) The cutting needs to be 20 metres across. Using the result of (c) calculate the volume of soil to be removed.
Provide complete and step by step solution for the question and show calculations and use formulas.