Question 1. Consider the market for watches where the market demand and market supply curves are given by the equations below where P is the price per watch unit and Q is the quantity of watches measured in watch units (that means that you can have fractional units of watches in your answers!):
Market Demand: P = 500 – 10Q
Market Supply: P = 100 + (10/3)Q
a) Given the above information, find the equilibrium price and quantity in this market. Then calculate the value of consumer surplus (CS) and producer surplus (PS).
b) Draw a graph illustrating this market and in your graph identify the equilibrium price, equilibrium quantity, all intercepts, the area that is CS and the area that is PS.
Suppose that the government in this economy decides to impose an excise tax of $50 per watch on producers of watches.
c) Given this excise tax, write an equation that represents the supply curve in this market now that producers have this new additional cost.
d) Given this excise tax, find the new price consumers will pay for a watch in this market, the new price producers will receive for a watch in this market once they have met their legal obligation to the government to remit the excise tax, and the new equilibrium quantity of watches that will be sold in this market.
e) Given this excise tax, calculate the value of consumer surplus with the tax (CSt), producer surplus with the tax (PSt), tax revenue the government receives from implementing the tax, consumer tax incidence (CTI), producer tax incidence (PTI), and the deadweight loss (DWL) due to the implementation of this excise tax.
f) Draw a graph illustrating this market and this excise tax. In your graph identify the price consumers pay for a watch now that the tax has been implemented, the price producers receive once they have paid the government the excise tax, the area of CSt, the area of PSt, the area of CTI, the area of PTI, and the area of DWL.
g) Suppose that the government decides it wants to implement an excise tax in this market so that consumption of watches fall to 12 units. Calculate the size of the excise tax that would be needed (assume that there is no initial excise tax) for the government to accomplish this goal. Show how you found your answer.
h) As the size of the excise tax increases, what happens to the area of DWL? Provide a verbal explanation and assume that the demand curve is downward sloping and the supply curve is upward sloping.
Question 2. Consider a small, closed economy whose market for cups is described by the following demand and supply equations where P is the price per cup and Q is the quantity of cups:
Domestic Demand: P = 10 – (1/20)Q
Domestic Supply: P = (1/80)Q
a) Assume this market is a closed market. Find the equilibrium price, equilibrium quantity, the value of consumer surplus (CS), and the value of producer surplus (PS). Show your work. Suppose this economy opens the cup market to trade and that the world price is $6 per cup.
b) Verbally explain whether this small economy will import or export cups given this information.
c) Now, provide a numerical value for your answer in (b). Make sure you show how you found your answer.
d) Suppose this market is still open to trade. Calculate the value of CS in the domestic economy when this market is open to trade (CStrade) and the value of PS in the domestic economy when this market is open to trade (PStrade).
e) Economists state that “trade is beneficial but has distributional consequences”. Explain this statement using your calculations in this problem as proof to support this statement.
Question 3. Consider a small economy whose market for cups is described by the following demand and supply equations where P is the price per cup and Q is the quantity of cups:
Domestic Demand: P = 10 – (1/20)Q
Domestic Supply: P = (1/80)Q
Suppose this economy opens the cup market to trade and that the world price is $1 per cup.
a) Given this information and assuming that this domestic economy opens its cup market to trade, find the value of imports, value of exports, value of consumer surplus with trade (CStrade), value of producers surplus with trade (PStrade), and the value of total surplus with trade (TStrade). Explain how you found your answers.
Suppose a tariff of $0.50 per cup is imposed on this good by the domestic economy’s government.
b) Given this tariff, find the values of the following items. Show how you found your answers.
Number of imports with tariff = ________
Number of exports with tariff = ________
Government tariff revenue = _________
CStariff = _______
PStariff = ________
DWL with tariff = _______
c) From the perspective of this domestic economy analyze the impact of this tariff. Who benefits from the tariff and how do they benefit? Who loses from the imposition of the tariff and what is their loss?
Question 4. Consider a small economy whose market for computers is described by the following demand and supply equations where P is the price per computer and Q is the quantity of computers:
Domestic Demand: P = 1000 - 10Q
Domestic Supply: P = 200 + 10Q
The world price is $300 for a computer.
a) Suppose this isinitially a closed economy. Find the equilibrium price and equilibrium quantity of computers in this closed economy.
b) Now, suppose this economy opens its computer market to trade. What will be the price of a computer given this decision? Calculate the number of computers produced by domestic producers, the number of computers demanded by domestic consumers, the number of imported computers into this economy, and the number of exported computers from this economy. Show how you found your answers. Suppose the government in this small domestic economy imposes an import quota of 40 computers in this market once it is open to trade.
c) Given this import quota and the provided information, find the values for the following (make sure you explain how you got your answer!). Hint: you might find it helpful to draw a graph to guide your work.
Price with the import quota = ________
Quantity of Computers demanded domestically with the import quota = ______
Quantity of Computers supplied domestically with the import quota = ________
DWL due to the imposition of the import quota = _________
License Holder Revenue with the imposition of the import quota = ______
Question 5. It is Thanksgiving and Uncle Henry and Rooster have gotten into a heated argument (this happens every year!). Rooster just got hired in an entry-level management position where he will earn $45,000 during his first year of employment. Uncle Henry is long retired but he is busy reminiscing about the “good old days” and he insists that life was much tougher for him when he was starting out. In fact he states that his first job paid him on $2500 a year. Uncle Henry got this job in 1948 while Rooster got his
job in 2013 (the 2014 data weren’t available last Thanksgiving).
a. Rooster argues that Uncle Henry is making a serious error in his argument. Write an explanation about what you think Uncle Henry’s error is.
Rooster goes to the computer and finds the Bureau of Labor Statistics site that provides him with the Consumer Price Index for 1948, 2013, and for the base year 1983.
Year CPI with base year 1983
1948 24.1
1983 100.00
2013 232.96
b. Given the above data, what scale is the CPI measured on?
c. Compute the following table’s missing values using 1983 as the base year. Make sure you show the work you did to get the missing values. Round your answer to the nearest whole number.
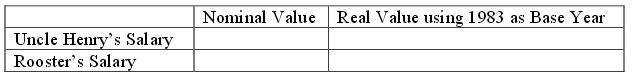
d) Compute the following table’s missing values using 2013 as the base year. Make sure you show the work you did to get the missing values. Round your answer to the nearest whole number.
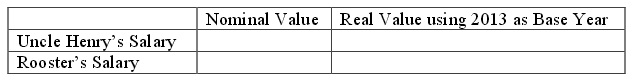
e) Uncle Henry contends that Rooster’s salary is 18 times greater than his starting salary was. Thus, Uncle Henry concludes that Rooster has it much easier than Uncle Henry did when he was first starting out.
Analyze this argument and provide some clarity for these two relatives! (If you need to in your answer, round any calculations to two places past the decimal.)
6. Consider the following demand curve for widgets where P is the price per widget and Q is the quantity of widgets.
Demand: Q = 1000 – 2P
a) Fill in the following table using the above information.
P Q Total Revenue = TR
$0
20
80
100
200
320
440
500
b) In your own words describe what happens to total revenue if the price of this good goes from $100 to $120. In your answer make sure you include references to the price and quantity effects.
c) What is the maximum total revenue that could be earned given this demand curve and holding everything else constant? Assume that the supplier is free to set any price they want and their goal is to set their price to maximize their total revenue.
Note: this price may not be in the table you just filled in!
Question 7. Consider the following market demand and market supply curves for pencils where P is the price per pencil and Q is the quantity of pencils.
Demand: P = 2 – (1/100)Q
Supply: P = 0.2 + (1/200)Q
a) Given the above information find the equilibrium price and quantity in this market. Show your work.
b) Calculate the point elasticity of demand at equilibrium. Provide the general formula and show your calculations. Is demand elastic or inelastic? Explain your answer. Given this answer, will producers enhance their total revenue by increasing or by decreasing the price they charge for pencils?
c) Calculate the point elasticity of supply at equilibrium. Provide the general formula and show your calculations. Is supply elastic or inelastic? Explain your answer.
d) Suppose the price increases to $1.00. Using the arc elasticity formula calculate the price elasticity of demand between the initial equilibrium and this new point on the demand curve. Provide the general formula and show your work. Is demand inelastic or elastic? Explain your answer.