1. (a) Convert the following units:
(i) 300 K = °C
(ii) 25°C = K
(iii) 1 bar gauge = bar absolute
(iv) 1 kN m-2= bar
(v) 2 bar = Nm-2
(take atmospheric pressure = 1 bar)
(b) What are the units of the following?
(i) specific internal energy
(ii) specific volume
(iii) work.
2. A gas at a pressure of 2 bar occupies a volume of 0.2 m3. If the temperature of the gas is 25°C and the characteristic constant for the gas is 0.35 kJ kg-1K-1, calculate :
(a) the mass of the gas
(b) the pressure of the gas after it is compressed isothermally to half of its original volume. 3. 0.5 kg of a gas is held in a rigid container of volume 0.25 m3 at a temperature of 20°C. 20 kJ of heat energy is required to increase the temperature of the gas to 60°C.
When the same mass of gas is heated at constant pressure, 30 kJ of heat energy is required to create the same temperature rise. Calculate:
(i) the specific heat capacities of the gas at constant volume and constant pressure
(ii) the initial pressure of the gas
(iii) the change in volume of the gas when it was heated at constant pressure.
4. In a closed system a gas is compressed from a pressure of 3 bar absolute to a pressure of 5 bar absolute, and its volume changes from 0.9 m3 to 0.6 m3 according to the law p1V1n = p2V2n. Calculate:
(a) the value of the index n
(b) the work done, stating whether it is done on the system or on the surroundings. In the multi-choice questions that follow, more than one of the options may be true.
5. When a thermodynamic system undergoes a cycle
(i) the internal energy at the end of each cycle is zero
(ii) the internal energy of the system is always increasing
(iii) the internal energy of the system is always decreasing
(iv) there is no net change in internal energy in the system.
Briefly explain your answer.
6. A heat pump
(a) can deliver more heat to the hot reservoir than work delivered to it from the surroundings
(b) can deliver more heat to the hot reservoir than that taken from the cold reservoir
(c) must take more heat from the cold reservoir than it delivers to the hot reservoir
(d) must have a coefficient of performance of less than one.
Briefly explain your answer.
7. Calculate the maximum theoretical thermal efficiency of a heat engine operating between heat reservoirs at 500°C and 50°C.
8. State the second law of thermodynamics. 9. In a quasistatic process involving heat transfer the temperature difference between the system and surroundings is always
a. finite
b. infinitesimal
c. zero
d. changing.
Briefly explain your answer.
10. The Carnot cycle:
a. consists of polytropic processes
b. will convert all of the available heat energy into work
c. establishes the maximum theoretical efficiency of a heat engine
d. consists of internally reversible processes only.
11. 0.8 kg of gas receives heat at constant pressure until its volume doubles. Calculate the change in entropy of the gas. For the gas take cp = 1.006 kJ kg-1K-1and cv = 0.717 kJ kg-1K-1.
12. An engine operating on the Otto cycle has a stroke length of 100 mm and a piston diameter of 50 mm. What is the maximum clearance
volume which will give an air standard efficiency of 60%?
13. A dual cycle operates with an air intake of 1 bar and 50°C. The compression ratio is 15:1 and the maximum pressures and temperatures reached in the cycle are 75 bar and 2200°C.
a. Sketch a p-V and T-S diagram for the cycle.
b. Calculate the pressure, specific volume and temperature at the end of each process in the cycle and complete the table below.
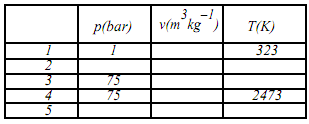
(c) Calculate the thermodynamic efficiency of the cycle.
14. A single cylinder diesel engine produces an output torque of 75 Nm when running at 1600 revs min-1. The indicated mean effective pressure in the cylinder is 5 bar and the piston diameter and stroke length are 150 mm and 250 mm respectively. Calculate :
a. the mechanical efficiency of the engine
b. the brake mean effective pressure. 15. A compressor has a piston diameter of 500 mm and a stroke length of 600 mm. If the clearance volume is 0.01 m3, the inlet pressure is 100 kN m-2, the delivery pressure is 500 kN m-2 and the index of compression is 1.3, calculate:
a. The volumetric efficiency of the compressor
b. If the pressure ratio is doubled, what will the new clearance volume need to be in order that the volumetric efficiency is not reduced?
16. Compressed air at a pressure of 10 bar is used at the rate of 0.2 m3 min-1. If the air is compressed from a pressure of 1 bar and temperature of 22°C at an index of compression of 1.26, calculate:
(i) the required power input to the compressor
(ii) the isothermal efficiency of the polytropic compression
(iii) the temperature of the air after the polytropic compression.
17. Air is to be compressed from a pressure of 1 bar and a temperature of 20°C to a pressure of 15 bar in a two stage compressor with intercooling. After intercooling the air temperature returns to 20°C and the polytropic index of compression is 1.28. If the input power per stage of compression is 2 kW, calculate:
(a) The volumetric flow rate of free air (at 1 bar and 20°C) in m3s-1.
(b) The power required if the same compression was carried out in one stage isothermally.
(c) Represent the isothermal and two stage compressions on a p-V diagram, indicating the work done for each process. 18. Given that the internal energy of water at 55 bar pressure is 1178 kJ kg-1 and that the specific volume of water at this pressure is 0.13.10-2m3kg-1, calculate the specific enthalpy of water at 55 bar pressure.
19. (a) Superheated steam at a pressure of 40 bar and a temperature of 500°C is supplied to the turbine of a Rankine cycle. If the condenser pressure is 0.03 bar, find the thermal efficiency of the cycle. Neglect feed pump work.
a. If the isentropic efficiency of the steam turbine in part (a) above is 0.82, find:
i. the enthalpy of the steam discharged into the condenser and
ii. the thermal efficiency of the cycle.
20. Steam at 30 bar and 500°C enters a pass-out turbine at a rate of 18000 kg h-1. The bulk of this steam is expanded isentropically to a condenser pressure of 0.03 bar, but 32% is bled from the turbine at a pressure of 3 bar for process heating. If the condensate from the process heater is directly pumped back to the boiler, find:
(a) the power output from the turbine
(b) the rate of process heating
(c) the efficiency of the plant. 21. Sketch on a T - s diagram the following cycle for a gas turbine:
a. Isentropic compression
b. Heat taken in at constant pressure p1
c. Isentropic expansion through turbine 1 from pressure p1 down to intermediate pressure p3
d. Reheat at pressure p3
e. Isentropic expansion from pressure p2, through turbine 2, down to final pressure p2.