1. Elasticity
In “Jormungand”, Ko ko Hekmatyar is an arms dealer. Suppose she faces the market for Javelin ATGM missiles, and the demand is given by Px = 10,000 - 2Qx.
Q1) Using the midpoint method , calculate the price elasticity of demand when price changes from $8,000 to $6,000. To answer the following questions, please use the point elasticity formula . Suppose you’re hired by Koko as her chief economist.
Q2) Calculate the price elasticity of demand when price equals $7500. Should Koko raise or lower the price of ATGM to gain more revenue if the current price is $7500? Why?
Q3) Calculate the price elasticity of demand when Qx equals 3500. Should Koko raise or lower the price of ATGM to gain more revenue when Qx equals 3500? Why?
Q4) What is the optimal price of ATGM you should suggest to Koko for total revenue maximization? At the optimal price, what is the point elasticity of demand? How much is the maximized total revenue? Suppose that there exists another company HCLI, who sells FIM-92 Stingers. The price of FIM-92 Stingers can be represented as Py.
Q5) When the price of FIM-92, Py, decreases from $3000 to $1500, the demand for ATGM, Qx, also decreases from 6000 to 5000. What is the cross-price elasticity of demand equal to given this information? Are ATGM and FIM-92 substitutes or complements? Use the standard formula for percentage changes to compute this value.
2. Excise Tax
Consider the market for cigarettes in New York City and Los Angeles. Suppose the daily demand for cigarettes in NYC is given as Q d =1000 - 100P, and the demand in LA is Q d =900 - 200P. The market supply for the two markets is the same: Q s =100+200P. Assume these two markets are totally separated.
Q1) Find the equilibrium price and quantity in the cigarette market for both NYC and LA.
Q2) Calculate the point price elasticities of demand for both NYC and LA at equilibrium.
Q3) Calculate the point price elasticities of supply for both NYC and LA at equilibrium.
Suppose that the government plans to impose an excise tax on sellers of cigarettes in order to reduce the market quantity of cigarettes by 100 units in both the NYC market as well as the LA market (that is, a total reduction of 200 units).
Q4) Find the corresponding excise taxes for NYC and LA.
Q5) Calculate the government revenues , consumer tax incidence, producer tax incidence, and dead weight losses for both NYC and LA due to the imposition of this excise tax.
Q6) For both NYC and LA compare the fraction of the tax paid by consumers to the fraction of the tax paid by producers. Explain your results from the point of elasticity.
3. Elasticity and Total Revenue
The table below describes the demand of undergraduate students for chocolate muffins at a café on the campus. Suppose the students have a linear demand curve for muffins.
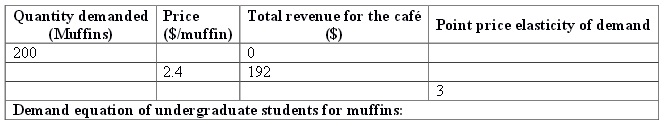
Q1) Complete the table above.
Q2) Find the price with which the café can maximize its revenue from undergraduate students. What's the point price elas ticity of demand at this maximum point for revenue? Now the demand equation for graduate students for chocolate muffins is Qd =200 - 40P, and the demand equation for faculty for chocolate muffins is P=10 - 0.05Q d .
Q3) Suppose that this café can charge different prices to different groups of customers. What are the maximum revenues from gradu ate students and faculty if the café charges different prices to the two groups ?
Q4) Suppose that the café cannot discriminate among those three groups of customers and that the market is only composed of these three groups of customers . In order to maximize the total revenue from all the customers, which price should this café charge? What's the maximum revenue if there is only one price for muffins? C alculate the point price elasticities of demand , respectively, for each group of customers at this single pri ce? Hint: these numbers will get a bit messy, but persevere
4. Real and Nominal Price
Suppose John Titor is a time traveler from a future year. He hopes to travel back in time to get one IBM 5100 which is unavailable in the future year that he lives in. He seeks to travel back in time to get this computer so that he can use it to fix a bug that threatens the computer system in the future. After several time travel trips back in time he has the following data about the IBM 5100 computer.

In addition, suppose there is a time travel rule that states that purchases at times other than the future time can only be made using real prices.
Q1) Examining the above table, what year is being used as the base year? That is, which year is the current dollar being used to measure the real price of the IBN 5100?
Q2) Fill in the missing values in the table.
Q3) Suppose John travels from 1975 to 1996 and when he travels he brings gold with him. This gold has a value of $20,000 in 1975 dollars . John knows that he does not have enough gold to buy the IBM 5100 in 1996 and he is trying to figure out how much additional nominal dollars (1996 dollars) he would need in order to have enough dollars to purchase one IBM 5100 in 1996. Can you help him figure out the additional nominal dollars he would need?
Q4) Suppose John has nominal income of $79,000 in 2010. Given this nominal income, which years could John travel back in time and be able to afford one IBM 5100? Suppose now we change the base year from 1975 to 2000. Assume that the nominal price of an IBM 5100 in each year in the table does not change.
Q5) What is the CPI now in 1975?
Q6) If the base year is 2000 for the CPI, then what is the real price of an IBM 5100 in 1975? John is also interested in how the CPI is calculated for different years. He has the following information.
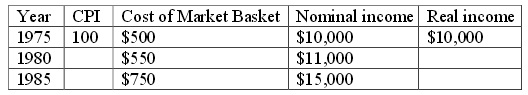
Q7) Please help John fill out the above table.
Q8) What is the inflation rate between 1975 and 1985?
Q9) Given t he table you computed in part (7), what is happening to consumer’s real income over this period of time? Does consumer welfare as measured by real income improve, stay the same, or decline between 1975 and 1985?
5. Budget Constraint:
Consider an economy composed of two goods, X and Y. The prices producers receive for a unit of these goods are, respectively, $2 for X and $3 for Y. The consumer has income of $60 to spend on the two goods. (Hint: The following questions are independent fr om each other.)
Q1) Write the consumer's budget constraint. Graph the consumer's budget constraint with X on the horizontal axis and Y on the vertical axis.
Q2) Suppose there is a tax of $1/unit imposed on good X. Write the new budget constraint, and graph it.
Q3) Suppose there is a subsidy of $1/unit bestowed on good Y. Write the new budget constraint, and graph it.
Q4) Suppose there is a tax on both goods that raises the price of each good by 50%. Write the new budget constraint, and graph it.
Q5) Suppose there is a lum p - sum tax of $10 levied on the consumer. That is, suppose the individual must pay the government $10 due to this new tax . Write the new budget constraint, and graph it.