Assignment:
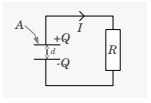
1. Consider a charged capacitor with charge ±Q on both plates, connected to a resistor R. The capacitor will start to discharge through the resistor. Assume a parallel-plate capacitor geometry, with plates of both surface area A and separation d.
(a) Calculate the initial energy density in the capacitor at t = 0, expressed in terms of the strength of the electric field in the capacitor E. And use as ‘volume' the volume of the insulating space Ad.
(b) Calculate the current as a function of time, starting at t = 0. You will need to calculate Q(t) first, it is not given.
(c) Calculate the time dependence of the power dissipated in the resistor from t = 0 to t = ∞ and integrate that to get the total energy dissipated.
(d) Compare answer 1a to 1c
2. Consider the circuit diagram on the right, with R = 1?, V 1 = 5 V, V 2 = 10 V, V 3 = 12 V. Calculate the current in the second branch, marked ‘I?'
