Calculate the angle between the two vectors

Question

Assignment:

Problems:

1) Given three vectors; F₁ = 35, F₂ = 40, F₃ = 80 (see the diagram for the angles), find the resultant vector of all three using the component method.

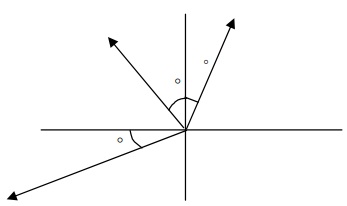
2) Repeat Problem 1 using unit vectors. Make sure you write out each vector using unit vectors first and show ALL of your work.

3) Using the vectors F₁ and F₂ from Problem 1, find the following, leaving your answers in unit vector notation.

a) F₁ - F₂

b) 2F₂ - F₁

c) F₁ + F₂

d) F₂F₁

e) F₂ (F₁ + F₂) / F₁

4) For the vector A = - 8j + 4k, find its magnitude and direction. Also state in what plane the vector resides.

5) Given the vector: A = 3i + 4j, find any three vectors B that also lie in the xy plane and have the property that A = B but A ≠ B. Write out these vectors with unit vector notation.

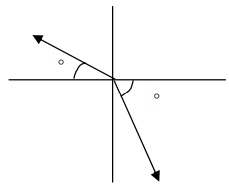
6) Given two vectors; S = 2.5, R = 4.2, (see the diagram for the angles), find the dot product using (a) the magnitudes and (b) unit vector notation.

7) Calculate the angle between the two vectors given by: b = 3i - 2j - 8k , c = i - 5j + 4k.

8) Determine if the following vectors are perpendicular to each other.

a) P = 3i - 2j + k , Q = 4i + 9j + 6k.

b) D = i + 2j - 5k , F = 2i - 7j + k.

9) Determine the vector product of each set of vectors.

a) P = 3i - 2j + k , Q = 4i + 9j + 6k.

b) D = i + 2j - 5k , F = 2i - 7j + k.