Assignment:
Problems:
1) Skid and Mitch are pushing on a sofa in opposite directions with forces of 530 N and 370 N respectively. The mass of the sofa is 48 kg. The sofa is initially at rest before it accelerates. There is no friction acting on the sofa. (a) Calculate the acceleration of the sofa. (b) What
velocity does the sofa have after it moves 2.5 m? (c) How long does it take to travel 2.5 m?
2) You have three force vectors acting on a mass at the origin. Use the component method we covered in lecture to find the magnitude and direction of the resultant force acting on the mass.
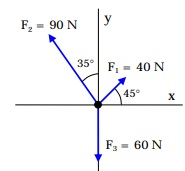
3) You have three force vectors acting on a mass at the origin. Use the component method we covered in lecture to find the magnitude and direction of the resultant force acting on the mass.

4) A bowling ball rolls off of a table that is 1.5 m tall. The ball lands 2.5 m from the base of the table. At what speed did the ball leave the table?
5) Skid throws his guitar up into the air with a velocity of 45 m/s. Calculate the maximum height that the guitar reaches from the point at which Skid lets go of the guitar. Use energy methods.
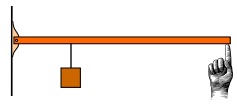
6) A beam of mass 12 kg and length 2 m is attached to a hinge on the left. A box of 80 N is hung from the beam 50 cm from the left end. You hold the beam horizontally with your obviously powerful index finger. With what force do you push up on the beam?
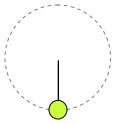
7) The tennis ball of mass 57 g which you have hung in your garage that lets you know where to stop your car so you don't crush your garbage cans is entertaining you by swinging in a vertical circle of radius 75 cm. At the bottom of its swing it has a speed of 4 m/s. What is the tension in the string at this point?
8) Derivatives:
a) Given: y = (4x + L)(2x2- L), find dy/dx .
b) Given: y = 1n(2x + L/2x - L), find dy/dx.
9) Intergrals:
a) Given: -45°∫45° (kλcos θ/r) dθ, evaluate.
b) Given: 0∫R 2kxr/(r2 + x2)3/2) dr, evaluate.
10) A plastic rod has a charge of -2.0 μC. How many electrons must be removed so that the charge on the rod becomes +3.0μC?
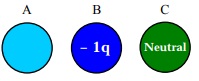
11) Three identical metal spheres, A, B, and C initially have net charges as shown. The "q" is just any arbitrary amount of charge. Spheres A and B are now touched together and then separated. Sphere C is then touched to sphere A and separated from it. Lastly, sphere C is touched to sphere B and then separated from it. (a) How much charge ends up on sphere C? What is the total charge on the three spheres (b) before they are allowed to touch each other and (c) after they have touched? (d) Explain the relevance of the answers to (b) and (c).
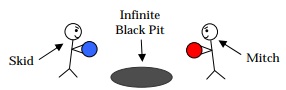
12) Skid of 40 kg and Mitch of 60 kg are standing on ice on opposite sides of an infinite black pit. They are each carrying neutral massless spheres while standing 8 m apart. Suppose that 3.0 x 1015 electrons are removed from one sphere and placed on the other. (a) Calculate the magnitude of the electrostatic force on each sphere. Are the forces the same or different? Explain. (b) Calculate the magnitude of the accelerations for Skid and Mitch at the moment they are 8 m apart. Are they the same or different? Explain. (c) As Skid and Mitch move closer together do their accelerations increase, decrease, or remain the same? Explain.
13) An electron travels in a circular orbit around a stationary proton (i.e. a hydrogen atom). In order to move in a circle there needs to be a centripetal force acting on the electron. This centripetal force is due to the electrostatic force between the electron and the proton. The electron has a kinetic energy of 2.18 x 10-18 J. (a) What is the speed of the electron? (b) What is the radius of orbit of the electron?
14) Three charges are arranged as shown. From the left to the right the values of the charges are 6 μC, - 1.5 μC, and - 2 μC. Calculate the magnitude and direction of the net electrostatic force on the charge on the far left.
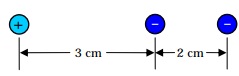
15) For the same charge distribution of Problem #5, calculate the magnitude and direction of the net electrostatic force on the charge on the far right.
16) Two charged spheres are connected to a spring as shown. The unstretched length of the spring is 14 cm. (a) With Qa = 6 μC and Qb = - 7 μC, the spring compresses to an equilibrium length of 10 cm. Calculate the spring constant. (b) Qb is now replaced with a different charge Qc. The spring now has an equilibrium length of 20 cm. What is the magnitude of the charge Qc? (c) What is the sign of Qc? How do you know this?

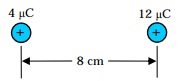
17) The two charges above are fixed and cannot move. Find the location in between the charges that you could put a proton so that the proton would have a net force of zero.
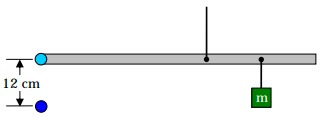
18) Three charges are fixed to an xy coordinate system. A charge of -12 μC is on the y axis at y = +3.0 m. A charge of +18 μC is at the origin. Lastly, a charge of + 45 μC is on the x axis at x = +3.0 m. Calculate the magnitude and direction of the net electrostatic force on the charge x = +3.0 m.
19) Four charges are situated at the corners of a square each side of length 18 cm. The charges have the same magnitude of q = 4 μC but different signs. See diagram. Find the magnitude and direction of the net force on lower right charge.
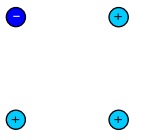
20) For the same charge distribution of problem #10, find the magnitude and direction of the net force on upper right charge.
21) All the charges above are multiples of "q" where q = 1μC. The horizontal and vertical distances between the charges are 15 cm. Find the magnitude and direction of the net electric force on the center charge.
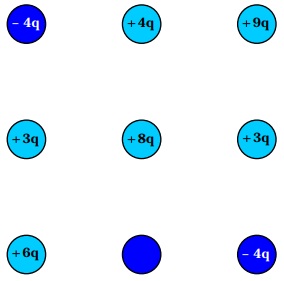
22) Use the same charge distribution as in problem #12 but change all even-multiple charges to the opposite sign. Find the magnitude and direction of the net electric force on center charge.
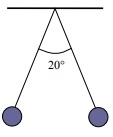
23) Two small metallic spheres, each of mass 0.30 g, are suspended by light strings from a common point as shown. The spheres are given the same electric charge and it is found that the two come to equilibrium when the two strings have an angle of 20° between them. If each string is 20.0 cm long, what is the magnitude of the charge on each sphere?
24) A meter stick of 15 kg is suspended by a string at the 60 cm location. A mass, m, is hung at the 80 cm mark. A massless charged sphere of + 4 μC is attached to the meter stick at the left end. Below this charge is another charge that is fixed 12 cm from the other when the meter stick is horizontal. It has a charge of - 4 μC. Calculate the mass, m, so that the meter stick remains horizontal.