For Problems 1–4, suppose Johnson & Johnson and the Walgreen Company have expected returns and volatilities shown below, with a correlation of 22%.
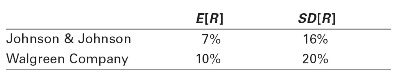
1.Calculate (a) the expected return and (b) the volatility (standard deviation) of a portfolio that is equally invested in Johnson & Johnson’s and Walgreen’s stock.
2.For the portfolio in Problem 22, if the correlation between Johnson & Johnson’s and Walgreen’s stock were to increase,
a. Would the expected return of the portfolio rise or fall?
b. Would the volatility of the portfolio rise or fall?
3.Calculate (a) the expected return and (b) the volatility (standard deviation) of a portfolio that consists of a long position of $10,000 in Johnson & Johnson and a short position of $2000 in Walgreen’s.
4.Using the same data as for Problem 1, calculate the expected return and the volatility (standard deviation) of a portfolio consisting of Johnson & Johnson’s and Walgreen’s stocks using a wide range of portfolio weights. Plot the expected return as a function of the portfolio volatility. Using your graph, identify the range of Johnson & Johnson’s portfolio weights that yield efficient combinations of the two stocks, rounded to the nearest percentage point.