Question 1:
Staley is at a carnival. The following table gives the total utility that Staley gets from eating bratwursts at the carnival.
Number of Bratwursts Total Utility Marginal Utility
0 0 ---
1 6
2 12
3 17
4 19
5 20
6 19
1) Calculate the marginal utility of eating each unit of bratwurst.
2) If the bratwursts are free, how many bratwursts would Staley choose to eat?
Suppose there is also free ice cream at the carnival. The following table gives the marginal utility of eating ice cream. Calculate the total utility of eating each unit of ice cream.
Ice Cream Total Utility Marginal Utility
0 0 ----
1 5
2 4
3 3
4 2
5 1
6 0
3) Suppose now that Staley has to pay for the bratwursts and ice cream. Each bratwurst cost $2 and each ice cream costs $4. Staley’s income equals $16. How many bratwursts and ice cream would Staley consume?
4) Suppose the price of ice cream decreases to $2. Holding everything else constant, how many bratwursts and ice cream would Staley consume?
5) Assume Staley’s demand function for ice cream is linear. Using the results from part 3) and part 4), derive Staley’s demand function.
Question 2: Budget Lines and Indifference Curves
A. Suppose Tayler has an income of $480 to buy two goods: pizza and textbooks. The price of a slice of pizza is $2, and the price of a textbook is $80.
1) Draw Tayler’s budget line (BL1) given her income is $480. (Put textbooks on the X-axis and pizza on the Y-axis.) Assume Tayler’s utility function is U(x,y)=xy2 (x is the purchase of the number of textbooks and y is the purchase of the number of slices of pizza). Tayler’s marginal utility of consuming a slice of pizza is 2xy. Tayler’s marginal utility of consuming a textbook is y2.
2) Derive Tayler’s Marginal Rate of Substitution of textbooks for pizza.
3) Derive Tayler’s budget line.
4) Find the optimal consumption point. What is Tayler’s utility? On your graph draw the indifference curve (IC1).
5) Suppose the price of textbooks decreases to $40. Find the optimal consumption point given this new price for textbooks. Draw the new budget line (BL2) and indifference curve (IC2) on your graph. Label the budget line and indifference curve clearly.
6) Given the price change described in part (4), if Tayler consumes optimally, how much income would be required for Tayler to obtain the same level of utility that she had in part (3)? After you calculate this compensated level of income, draw an imaginary budget line (BL3) using this new income and the new prices on your graph.
7) What is the substitution and income effect in textbook consumption due to the change in the price of textbooks? Illustrate the effect on your graph.
B. Sarah loves musicals. Sarah receives 15 units of utility for every Les Miserables performance she goes go. Sarah receives 10 units of utility for every Phantom of the Opera performance she goes to. Suppose Sarah’s income is $300. The price of either shows is $100 each. Assume that the utility from consumption of an additional unit of either good is constant.
1) Draw Sarah’s budget line (BL1) given her income is $300. (Measure Phantom of the Opera on the Y-axis and Les Miserables on the X-axis.)
2) Please find the utility maximization point and draw an indifference curve (IC1) through the utility maximization point on your graph.
3) Suppose the price of a Les Miserables performance increases to $200, draw Sarah’s new budget line (BL2) and find her new utility maximization consumption bundle on your graph.
4) Draw an imaginary budget line (BL3) parallel to the new budget line (BL2) and make it touch the initial indifference curve (IC1) at the lowest possible income level. Calculate and show the income and substitution effects in the consumption of Les Miserables performances due to the price increase.
C. Amanda is buying orange juice and vodka to mix drinks. She wants 1 bottle of vodka for every carton of orange juice. She does not like orange juice by itself and she does not like drinking straight vodka. The price of a cartoon of orange juice is $2, and the price of a bottle of vodka is $10.
1) Draw Amanda’s budget line (BL1) given her income is $36. (Put vodka on the X-axis and orange juice on the Y-axis.)
2) Please find the utility maximization point and draw an indifference curve (IC1) through the utility maximization point.
3) Suppose the price of a bottle of vodka increases to $16, draw Amanda’s new budget line (BL2) and find her new utility maximization consumption bundle.
4) Draw an imaginary budget line (BL3) parallel to the new budget line (BL2) and make it touch the initial indifference curve (IC1) at the lowest income level possible. Calculate and show the income and substitution effects on the consumption of vodka due to the price change in vodka.
Question 3: Production Cost I
The following table gives cost information for a firm. Assume that labor is paid a constant wage and capital is paid a constant price, i.e., our firm is a price-taker both in the labor and capital markets.
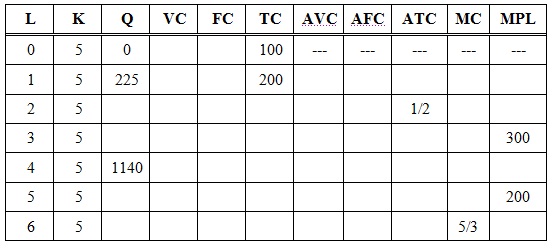
1) Suppose output is equal to zero. Why does the firm still incur costs in the short run when output is equal to zero? What is the fixed cost of this firm? What is the price of a unit of capital?
2) What is the wage rate?
3) Please complete the above table with specific numbers.
4) At what level of output and labor usage is AVC at its minimum?
5) At what level of output and labor usage is ATC at its minimum?
6) At what level of labor usage does the law of diminishing returns first occur?
7) According to the table, what is the minimum price for the firm to be willing to hire the fourth unit of labor? According to the table, what is the minimum price for the firm to be willing to hire the fifth unit of labor? What is the price range of the product if the optimal number of labor hired by the firm is 4?
Question 4: Perfect Competition
Suppose a perfectly competitive firm’s cost function is C(q) = 4q2 + 16. Marginal cost for the firm is given by MC = 8q.
1) Find equations for variable cost, fixed cost, average total cost, average variable cost and average fixed cost for this firm. Illustrate on a graph the firm’s average variable cost curve, average total cost curve, and marginal cost curve.
2) Find the outputs that minimize average total cost, average variable cost and average fixed cost.
3) What is the shutdown price for this firm?
4) What is the breakeven price for this firm?
5) If the price of the output is $32, how many units will this firm produce? What is the firm’s profit?
6) Suppose market demand for this good is given as P = 300 - Q and all firms in the market are identical. How many firms are active in the market in the long run?