Problem 1. Monopoly
In Madison, the University owns the rights to sell Badger Soccer tickets. The costs of providing these tickets is represented by TC = Q2 + 100 .
That is, each additional ticket costs 2Q to the university (MC = 2Q ) and the University rents the stadium from the lease holder for $100 per game. The demand for Badger Soccer tickets is given by: P = 300 – Q .
a) What is the equation for UW’s Badger Soccer ticket Marginal Revenue curve?
b) Draw the Demand Curve, Marginal Revenue Curve, and Marginal Cost Curve. What is the profit-maximizing production quantity of soccer tickets sold? What is the price of a Badger soccer ticket?
c) Compute the consumer surplus, producer surplus, DWL, and profits for the monopolist. Will the University continue to sell Badger Soccer tickets in the long run? Suppose the university is forced to behave as if there is perfect competition and charge P = MC
d) Repeat c) and d) with this MC regulation. e) Which regulation (No regulation, MC) do the consumers prefer? Which regulation does the University prefer? (Remember, if the university is forced to shut down or exit, surplus in the long run will be 0).
Problem 2. Price Discrimination
MG&E is a Natural Monopoly in Madison. They supply electricity (Q = megawatts) for a price (P) per megawatt. They have constant Marginal Costs and high fixed costs. In this case, TC = 10 Q + 200. MC = 10.
There are 2 groups of consumers in the market. The first group of consumers is composed of College Students. The second group of consumers is composed of Professors .
The demand for Electricity for College students is: P = 110 – Q
The demand for Electricity for Professors is: P = 150 – Q
Suppose MG&E is allowed to price discriminate and charge a different price to College Students and Professors.
a) What price and quantity of electricity will MG&E set for Students?
b) What price and quantity of electricity will MG&E set for Professors?
c) What are the total profits made by MG&E (remember, they only need to pay their Fixed Costs once)?
The Mayor of Madison decides to step in and not allow MG&E to price discriminate. Now, MG&E can only set one price.
d) What is the equation for the market demand that MG&E faces? (remember to write market demand with respect to quantities sold)
e) Find the new marginal revenue curve for each part of the demand curve (with respect to quantity). Graph the Demand curve, Marginal Revenue curve, and Marginal Cost curve.
f) What price will MG&E set to maximize profits now that they can only set 1 price?
Problem 3. Public Goods
The city of Madison is looking to build some new parks in the city. The city is comprised of two types of people: college students and families. The families would really enjoy having some new parks and their demand for the new parks is given by P = 24 – Q where Q is the number of new parks and P is the price per park. The students have very little free time because their econ 101 class is so time consuming and so they are unwilling to pay very much for new parks. The students ’ demand for new parks is given by P = 12 – 3 Q.
The total cost for a new park is given by the following: TC = 24 Q. This implies that the MC of a new park is MC = 24 .
a) If the students try to free-ride off the families and not contribute to the new parks, how many new parks will be provided if only the families pay for the new parks?
b) What is the total demand for the park? To find the market demand for a park, we need to VERTICALLY sum the demand curves. You fix the size (Q) of a park and see how much in total people are willing to pay for that size.
c) How much of the park will be built if both students and families contribute to the park (that is, there is no free riding)?
d) In order to implement this socially optimal outcome, you should find what each group pays to fund the quantity of new parks. How much per park do students pay and how much per park do families pay?
Hint: the total contribution by students and families must equal $24 per new park. Do you think this is a fair way to fund a public good? How do you think the government finds the willingness to pay for a public good?
Problem 4. Game Theory
Currently Bucky’s Candy Canes is the only producer of candy canes in Madison, with annual profits of 3 million dollars. A new producer, Golden Gopher Novelties, is contemplating entering the market in Madison. Bucky’s and Golden Gopher must decide simultaneously ( i.e., without knowing what the other firm will do) if they will sell Candy Canes in Madison next year . If Golden Gopher enters the market and Bucky’s remains in the market , Bucky’s will earn 1 million dollars in profits and Golden Gopher will lose 2 million dollars next year . If Golden Gopher enters the market and Bucky’s leaves, Golden Gopher will lose 1 million dollars next year.
If Bucky’s leaves the market, it will make 0 profits, regardless of whethe r Golden Gopher enters. Similarly, if Golden Gopher does not enter the market, it will make 0 profits, regardless of whether Bucky's remains in the market.
a) Use the information above to complete the following payoff matrix (it would be best if you drew this game in your homework rather than enter the numbers here):
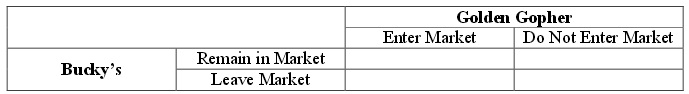
b) Does Bucky’s have a strictly dominant strategy? Explain your answer.
c) Does Golden Gopher have a strictly dominant strategy? Explain your answer.
d) What is the equilibrium outcome?
Problem 5. Externalities
Demand for education is characterized by the following equation: P = 120 – 2Q. The marginal private cost of education is P = 20 + 3Q. Production of education also creates a per unit positive externality equal to Q.
a) Suppose that the market is currently unregulated (i.e., the positive externality is not currently being corrected for in the market). What is output and price?
b) The marginal social cost (MSC) accounts for the marginal private cost of production as well as for the additional benefits received by the society. What is the MSC equation for production of education?
c) What is the socially optimal level of output and price?
d) Suppose that the government wants to achieve the socially optimal outcome. One way to do that is for the government to subsidize producers so that the firm’s marginal cost decreases by the amount of the positive externality. What subsidy per unit of output should the government impose ? What is the marginal cost of production a fter the subsidy ?