1. Long-run figures for the economy are reported in TABLE 1
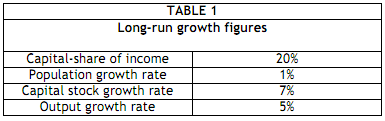
Assume that the country's production function is a constant-returns-to-scale Cobb-Douglas, Y = AKaLb, where Y is real national income, K is national capital stock and the total factor productivity is represented by A. Total population coincides with total workers and is denoted by L.
(a) compute the Solow residual
- state clearly/describe the formula you are using
- compute the mathematical result
Now consider some additional data provided reported in TABLE 2

(b) Determine the steady state level of ‘consumption per human-capital-augmented efficiency units of labour' (c')
- state clearly/describe the formula you are using
- compute the mathematical result
(c) Derive the growth rate of consumption-per-worker in the steady state (Δc/c)
- state clearly/describe the formula you are using
- compute the mathematical result
(d) Sketch graphically the representation of the steady state and illustrate what happens to the steady state when there is an increase in the rate of population growth. Explain your answer by discussing whether or not that is in line with the empirical evidence.
- state clearly/describe the graph you are using
- describe clearly the changes that occur to the graph and compare the final result with the initial situation
- give an economic intuition of the result, within the context of the model
- talk about the empirical evidence illustrated in class in relation to the effects of the exogenous change on the endogenous variables.