1. Construct an explicit deformation retraction of the torus with one point deleted onto a graph consisting of two circles intersecting in a point, namely, longitude and meridian circles of the torus.
2. Construct an explicit deformation retraction of Rn- {0} onto Sn-1
3. (a) Show that the composition of homotopy equivalences X→Y and Y→Z is ahomotopy equivalence X→Z . Deduce that homotopy equivalence is an equivalencerelation.
(b) Show that the relation of homotopy among maps X→Y is an equivalence relation.
(c) Show that a map homotopic to a homotopy equivalence is a homotopyequivalence.
4. A deformation retraction in the weak sense of a space X to a subspace A is ahomotopyft:X→X such that f0= 11, f1(X) ⊂A, and ft(A) ⊂A for all t . Show that if X deformation retracts to A in this weak sense, then the inclusion mapA?Xis a homotopy equivalence.
5. Show that if a space X deformation retracts to a point x ∈X, then for each neighborhood U of x in X there exists a neighborhood V ⊂U of x such that the inclusion map V? U is nullhomotopic
6. Show that a retract of a contractible space is contractible.
7. 10. Show that a space X is contractible iff every map f :X→Y , for arbitrary Y , is nullhomotopic. Similarly, show X is contractible iff every map f: Y→X is nullhomotopic.
Group 2 :
1. Show that composition of paths satisfies the following cancellation property: If
i. f0.g0?f1.g1 and g0?g1 then f0?f1.
2. Show that the change-of-basepoint homomorphism βhdepends only on the homotopyclass of h.
3. For a path-connected space X, show that π1(X) is abelian iff all basepoint-changehomomorphismsβhdepend only on the endpoints of the path h.
4. Show that for a space X, the following three conditions are equivalent:
(a) Every map S1→X is homotopic to a constant map, with image a point.
(b) Every map S1→X extends to a map D2→X.
(c) π1(X,x0) = 0 for all x0∈X.
5. Does the Borsuk-Ulam theorem hold for the torus? In other words, for every mapf :S1×S1→R2 must there exist (x,y) ∈S1×S1 such that f (x,y) = f (-x,-y)?
6. From the isomorphism π1(X×Y, (x0,y0)) ≈ π1(X,x0)×π1(Y,y0) it follows that
7. loops in X×{y0} and {x0}×Y represent commuting elements of π1(X×Y, (x0,y0).Construct an explicit homotopy demonstrating this.
8. If X0 is the path-component of a space X containing the basepointx0 , show thatthe inclusion X0?X induces an isomorphism π1(X0,x0)→π1(X,x0).
9. Show that every homomorphism π1(S1)→π1(S1) can be realized as the inducedhomomorphism ?∗of a map ?: S1→S1 .
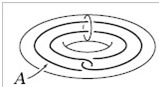
10. Show that there are no retractions r :X→A in the following cases:
(a) X = R3 with Aany subspace homeomorphic to S1 .
(b) X = S1×D2 with A its boundary torus S1×S1 .
(c) X = S1×D2 and A the circle shown in the figure.
(d) X = D2∨D2 with A its boundary S1∨S1 .
(e) X the Möbius band and Aits boundary circle.