--%>
Assignment Help - homework help

Describe First Order Rate Equation

The integrated forms of the first order rate equations are conveniently used to compare concentration time results with this rate equation.

Rate equations show the dependence of the rate of the reaction on concentration can be integrated to give expressions for the dependence of the concentrations on time. We generally use the integrated rate equation that is obtained to deduce the order of a reaction.

A first order reaction is one for which, at a given temperature, the rate of the reaction depends only on the first power of the concentration of a single reacting species. If the concentrations of this species is represented by c (for solutions, the units of moles per litre are ordinarily used), and if the volume of the system remains essentially constant during the course of the reaction, the first order rate equation can be written

-dc/dt = kc

The rate of constant k is then a positive quantity and has the units of the reciprocal of time.

Integrated rate equation: the experimental results obtained in a study of the rate of a reaction are usually values of c or some related to c at various times. Such data can best be compared with the integrated form of the first order rate equation. If the concentration at time t = 0 is c0, and if at some later time t the concentration has fallen to c, the integration gives

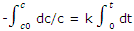
481_First order reactions.png 

With -In (c/c0) = In (c0/c), the integration can be written as

In c0/c = kt

Sometimes a more convenient form is

In c = -kt + In c0

A reaction can therefore be said to be first order if a plot of In (c0/c) or In c versus t gives a straight line. If a straight line is obtained, the slope of the line can be used to give the value of the rate constant k. an alternative to this graphical procedure is the calculation of a value of kfrom the individual measurements of c at the various times t, for example. The reaction is classified as first order if all the data lead to essentially the same values for k, that is, if it is satisfies with k as a constant.

Example: the rate of conversion of tert-butyl bromide to tert-butyl alcohol, (CH3)3CBr + H2O 1598_First order reactions1.png (CH3)3COH + HBr, has been studied and some concentration-time results are found in table given below. Verify that the reaction is first order, and deduce the values of the rate constant at the two temperatures.

Solution: from the data at each temperature we calculate In (c0/c) values. Then the graphical display shows a plot of In (c0/c) versus t is constructed. The straight lines, each going through the origin show that at both temperatures the data conform to the integrated first order relation. The slopes give the values of the rate constants 

K = 0.00082 min-1 = 0.137 × 10-4 s-1 [25°C]    

K = 0.0142 min-1 = 2.37 × 10-4 s-1 [50°C]    

Concentration of tert butyl bromide as a function of time for the reaction (CH3)3CBr + H2O 1598_First order reactions1.png (CH3)3COH + HBr in a 10% water, 90% acetone solvent

At 25°C  At 50°C
Time, h (CH3)3CBr, Mol L-1 Time, min (CH3)3CBr, Mol L-1
0 0.1039 0 0.1056
3.15 0.0896 9 0.0961
6.20 0.0776 18 0.0856
10.0 0.0639 27 0.0767
13.5 0.0529 40 0.0645
18.3 0.0353 54 0.0536
26.0 0.0270 72 0.0432
30.8 0.0207 105 0.0270
37.3 0.0142 135 0.0174
43.8 0.0101 180 0.0089

   Related Questions in Chemistry

  • Q : Units of Measurement Unit of

      Unit of measurement- These are also some systems for units:      (1) 

    		•
  • Q : Explain the process of adsorption of

    The extent of adsorption of a gas on a solid adsorbent is affected by the following factors: 1. Nature of the gas Since physical adsorption is non-specific in nature, every gas will get adsorbed on the

    		•
  • Q : Molecular Properties Symmetry Molecular

    Molecular orbitals and molecular motions belong to certain symmetry species of the point group of the molecule.Examples of the special ways in which vectors or functions can be affected by symmetry operations are illustrated here. All wave functions soluti

    		•
  • Q : Mole fraction Give me answer of

    Give me answer of following question. The sum of the mole fraction of the components of a solution is : (a) 0 (b) 1 (c) 2 (d) 4.

    		•
  • Q : Product of HCl Zn Illustrate  the

    Illustrate  the product of HCl Zn?

    		•
  • Q : Molar conductance what is the molar

    what is the molar conductance of chloropentaamminecobalt(III) chloride?

    		•
  • Q : Dipole moment of chloro-octane Describe

    Describe the dipole moment of chloro-octane in brief?

    		•
  • Q : Molality of a glucose solution What

    What will be the molality of a solution containing 18g of glucose (having mol. wt. = 180) dissolved in 500g of water: (i) 1m  (ii) 0.5m  (iii) 0.2m  (iv) 2m

    		•
  • Q : Molar mass Select the right answer of

    Select the right answer of the question. Which is heaviest: (a)25 gm of mercury (b)2 moles of water (c)2 moles of carbon dioxide (d)4 gm atoms of oxygen

    		•
  • Q : Question based on vapour pressure and

    Benzene and toluene form nearly ideal solutions. At 20°C, the vapour pressure of benzene is 75 torr and that of toluene is 22 torr. The parial vapour pressure of benzene at 20°C for a solution containing 78g of benzene and 46g of toluene in torr is: (a) 50 (b)

    		•